Dynamical Symmetries: Autopoietic ArchitectureThe Areas of Mathematical Synthesis Between Complexity, (edge of) Chaos Theory , Fractal Geometry and the Golden Mean: leading to an argument for an Autocatalytic Architectural approach based on emergent Self-Organised Criticality
Email: Nigel Reading <phimega@aol.com Ta panta rei --- all is flux
Heraclitus of Ephesus, sixth century B.C. Morphology is not only a study of material things and of the forms
of material things, but has its dynamical aspect ... in terms of force,
of the operations of energy. This is a great theme. Boltzmann, writing
in 1886 on the second law of thermodynamics, declared that available energy
was the main object at stake in the struggle for existence and the evolution
of the world.
D'Arcy Thompson, On Growth and Form, 1917 ...to (in)form buildings with thematic meaning, they must convey
a gestalt, the whole must be more than the sum of the parts, and there
must also be an ambiguity and paradox immanent within that gestalt, as
a tension. (And quoting Heckscher on composition...) It is the taut composition
which contains contrapuntal relationships, equal combinations, inflected
fragments, and acknowledged duality's. It is the unity which maintains,
but only just maintains, a control over the clashing elements which compose
it. Chaos is very near, its nearness, but its avoidance, gives ...force.
Robert Venturi, Complexity and Contradiction in Architecture, 1966
The Universe is constantly seeking to lower it's energy (like a stream meandering down a valley, sometimes becoming rapids or cataracts), as it continually breaks it's initial higher (energy and dimensioned) symmetry. This symmetry-breaking can be pictured as bifurcating branches of probabilities, of chance and causality intertwined. The reason for this is that the higher energy/dimensions of the Universe's origin were also highly symmetrical, which paradoxically, means that they were also extremely unstable. Highly symmetrical objects are unstable ( imagine the beginning of the universe as being like you sitting on a perfect, highly polished sphere), and have a high probability for instability because any deviation by you from the north pole will have you rapidly slipping off. Here, gravitational instabilities quickly break your symmetrical position at the north pole and pull you to a lower gravitational potential energy state. So small initial deviations in unstable, far from equilibrium situations can lead to massive, even cosmological consequences. The Butterfly effect (also known as sensitive dependence on initial conditions), is literally, Universal. Ludwig Boltzmann is known to us as the first to provide a probabilistic, statistical interpretation of entropy. This is simply the tendency of everything in the Universe to cool to a minimum energy or temperature --- known as thermal equilibrium. The route to this second law of thermodynamics is via increasing disorder in the organisation of energy and matter. The current symmetry-breaking from the initial condition leads therefore, from a highly symmetrical, ordered and energetic state towards the opposite, an asymmetrical, disordered and lower energy one; from a low entropy Big Bang to a higher entropy present and future. The great paradox of the second law then, is the evident, increasingly complex, emergent and hierarchical order we see all about us. How is this ordered, structured information (expressed in constantly oscillating patterns of matter and energy) allowed to coalesce and persist from this tendency towards the random --- towards increasing entropy? Dynamical systems theory also deals with probability and can therefore allow us to synthesise thermodynamics and so-called "Chaos", (which is really a highly complex form of hierarchical, enfolded order that appears to be disorder). The really interesting area here though, is the entities at the transition zone between ordered, stable systems at equilibrium (maximum entropy) and "disordered" (but complex) and unstable Chaotic (minimum entropy) ones. According to the Nobel laureate Ilya Prigogine, these far from equilibrium dissipative systems locally minimise their entropy production by being open to their environments --- they export it in fact, back into their environments, whilst importing low entropy. Globally, overall entropy increase is nevertheless preserved, with the important caveat that the dissipative system concerned often experiences a transient increase (or optimisation) of its own complexity, or internal sophistication, before it eventually subsides back into the flux. This is known as the region of alternatively, Emergence, Maximum Complexity, Self-organised Criticality, Autopoiesis, or the Edge of Chaos. (Nascent science debates nomenclature routinely - and appropriately, in this case, the crucial point being that they are all different terms for essentially the same phenomena.) Lifeforms, ecosystems, global climate, plate tectonics, celestial mechanics, human economies, history and societies, even consciousness itself - all manifest this feedback-led, reflexive behaviour; they maximise their adaptive capacities by entering this region of (maximum) complexity on the edge of Chaos, whenever they are pushed far from their equilibrium states, thereby incrementally increasing their internal complexity, between occasional catastrophes. Remarkably, this transition zone is mathematically occupied by The Golden Mean. This ratio acts as an optimised probability operator, (a differential equation like an oscillating binary switch), whenever we observe the quasi-periodic evolution of a dynamical system. It appears in fact, to be the optimal, energy-minimising route to the region of maximum algorithmic complexity, and to be a basin of attraction for the edge of Chaos. In this review, we shall cover some demonstrations of this behaviour, and seek to understand its role. As far as architectural application is concerned, we must look at the temporal as well as the spatial, at how quite literally, the dynamics (of systems applications - functions) can inform the statics (forms) of building. The aesthetics of the banal imitation of some motif of fractal geometry is simply painting half the picture! From the Egyptians to the Greeks, Gothic to the Renaissance and the Modernists in the Western tradition, and especially in Hindu, Islamic, Buddhist and Meso-American aesthetics, fractal structures or rubrics (such as the regulating lines of Le Corbusier or his Modular based on the "Divine" Golden Mean), have shown that intuitively, the best architecture has understood and reproduced the true geometry of nature as more than just decoration - but as pure, optimal structure, that allows the thematic, historic and actual forces and loads being carried to be read as a tension, as a dynamic equilibrium - from the scaled arches of Roman aqueducts to the tiered flying buttresses at Chartres, to the contrapuntal three-pin arch of Grimshaw's Waterloo International in London and perhaps most appositely in recent times, the structures of Calatrava, Hopkins, Piano, Rogers and Foster; the purest architecture has been taken from nature's own template. Aristotle implied over two millennia ago that the proper investigation required was one of telos, the "final cause" of morphology, of form being the result of the processes that engendered it. His "final cause" of morphogenesis suggests an imperative behind any generative process that has often been interpreted as having theological (as well as teleological) connotations. Here, we shall take a more determinist route, in line with his mentor Plato's definition of the logos, as the "proportion" which was commensurate in square, which best squared the circle, or presented a unity that was more than the sum of its parts. It will be suggested that this imperative behind form (as static, precipitate matter resulting from dynamic flows of energy) is certainly nothing to do with the metaphysical, but simply the result of the way nature minimises energy waste (entropy production), also known as the principle of least action - and that one way of mathematically representing this behaviour appears to be analogous to the dynamical behaviour of the Golden Mean. So how does nature manifest this limiting principle in a way that still allows for the immense emergent complexity we see, or to put it another way, how can we demonstrate that least action acts as an attractor for Complexity and self-organising emergence, by symmetry-breaking to lower energy states, towards the edge of Chaos? All fractal forms, inert (clouds, landscapes, galaxy clusters) or animate (plants, animals), are self-similar scaled copies of an original; chaotic systems (climate, the solar system, the stock market) also always possess this fractal quality, but taken to the paradoxical extreme of having infinite trajectories within a finite boundary. To produce these forms, a recursive feedback regime must be operating. Feedback (encoding similarities) underlies the entire subject, and is the basis of the thesis research (undertaken at the Engineering, Computer Science and Architecture Faculties, University of Westminster) that underlies this review. This research began several years ago (during the degree at Kingston University), as an intuition that The Golden Mean, or Phi for short, (as a ratio) must have been fractal in nature. By extension, it seemed plausible that Phi may also have been embedded in higher dimensional, dynamical systems as an attractor of some kind, since complex dynamical systems always have a fractal temporal structure as they evolve over time. A major clue leading to the above interpretations can be seen in the fact that Phi is simultaneously both an arithmetic and geometric expansion of itself and One of the simplest possible kind. This immediately places it in both the linear (arithmetic progression) and non-linear (geometric progression) realms, and as an effective bridge, operating between the two. Virtually every aspect of fractal geometry and type of dynamical system
can be expressed by variations upon the simple quadratic iterator:
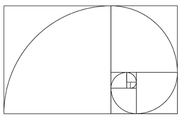
This leads us to the other cardinal feature of Phi. There is only one proportional division of One possible using two terms, with the third being One itself. From Euclid's ELEMENTS Book Five, Theorem Three (Alexandria, 3rd century B.C.): "A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the less." The Golden Mean then, is an archetypal fractal in that it preserves its relationship with itself (its inherent similarities under scaling are conformal symmetries - with topological consequences, that are invariant about themselves), in the most mathematically robust, economical but also elegant, way. It is analogia exemplified.
As we shall see, this reciprocal, squaring behaviour about One, or Unity, as it is more properly termed, is far from being mathematically trivial. All feedback loops deterministically involve the passage of time. The quadratic iterator is derived from Newton's differential calculus, and from a period when nature was seen as a mechanistic and time-reversible automaton. Recent science demonstrates that in fact it consists of both the above and irreversible processes, known as the entropy barrier, or the arrow of time. The Golden Mean can also be seen as mathematically (because of the above) the simplest and most stable way of communing or mediating between the two, as we shall see. As mentioned, iterated recursive loops (feedback), must occur over a certain time interval and have a beginning and an end. To give a crude example, this is analogous(derived from the Greek for "proportional action", better known to us as self-similarity) to the initiation of a system at the apex of a space-time light cone, and a progressive winding around its extruding surface (with cone length being the age of the system). The resulting two-dimensional spiral inscribed on the cone surface represents an extruded origin, being One, constantly growing over time but never changing its shape, an example of its optimum stability (for a logarithmic spiral, rotation and scaling are identical). The world line of this space-time system heads from the apex to the origin of the disk-base and is irreversible. However, there is mathematically, the reciprocal case: another world line heading in the opposite direction, at all times, producing together, a spindle form, with an infinitely thin pinch-point joining them and jointly, corresponding to a time-reversible scenario. This can be seen explicitly in the complex plane, as we shall see. This is how Phi mediates between the finite and infinite attractors, and allows for infinite co-dimensionality (scale and dimensional variations) and reversible/irreversible processes in its action, (all mathematical tools for probing natural behaviours, best thought of as self-consistent logical structures expressed in algebraic symbolic or geometric forms - in this case renormalisation operators, hamiltonian systems and eigen functions), as we shall develop upon. The feedback loop which describes Phi is an arithmetic linear operator, (like a binary switch: 0 or 1, off or on) representing the winding or rotation number of the inscribed spiral, which is conventionally represented as a multiple of 2pi;. This is superposed with its expansion ratio; 1 : 1.618 ... which is geometric (logarithmic) and therefore non-linear. This can be represented using the polygonal spiral that preserves the circle in half-radians (pi/2) in a manner reminiscent of the action of i = sqrt(-1), the imaginary number. (See below, re: the complex plane) Note that the natural logarithm e, i and pi are in the following relationship: e **(i pi) = -1. The linear is reversible (and finite) while the non-linear is not (and infinite --- being numerically irrational). Phi is in fact the most difficult irrational to approximate with rational numbers, making it the last KAM torus to collapse before the onset, or edge of Chaos. This quasi-periodic Phi toroid geometry is therefore paradoxically, the most stable (under perturbation), despite also being on the edge of Chaos. This is reminiscent of non-linearities damping dissipation in soliton behaviour. Solitons are persistent travelling waves which trade dissipative (resonant) effects off against inherent non-linearities, in a way that cancels each other out, (a flame being a simple example,). KAM (after Kolgomorov, Arnold, Moser), tori themselves are geometric models that have been used to solve many-body problems in physics, such as planetary dynamics: it has been suggested that the entire solar system is in this edge of Chaos scenario, as a global spatial/temporal entity - whilst allowing for local spatial/temporal chaotic instabilities, again known as resonances, which could be seen as fine adjustments to the overall dynamic, (such as the orbital gaps between the planets, moons and within ring systems; or the periodic ejection of asteroids from their usual orbits). This binary switching process, unique to Phi, which as noted, is reminiscent of the action of an eigen, rescaling or renormalisation operator, is analogous to the tossing of a coin, a chance or stochastic process, that allows a dynamical/probabilistic interpretation. This mathematics and resultant geometries, is also the paradigm for quantum mechanics, dynamical systems, and the relativistic (but with appropriate super-positions) or in other words, the maths of chance, for the linear Schrödinger probability equations of the quantum, or causality for the arbitrarily large velocities and mass/energies expressed by the non-linearLorentz transformations of the relativistic worlds. However, chance even enters the equations for relativity, since the Lorentz transformations that Einstein used to link space and time in Pythagorean relationships lead to singular points, where spacetime must end. A spacetime singularity could be seen as an infinity of sub-quantum, stochastic, hyper-chaotic events; whilst a mathematical singularity could be seen as an infinity of iterations of a certain logical structure, which approach infinity with arbitrary rapidity. The limits of the Big Bang and Black Holes are the defining singularities of our existence. Causality enters the quantum world only at its limits too: when the famous "collapse of the wavefunction" occurs, and a measurement is made (or interference occurs, from this or putative parallel universes), from the world of the classical-scale dynamical system - where deterministic Chaos (chance and causality in synthesis) is the rule. These Phi-type stochastic operators have been shown by
physicists to be super-stable mechanisms (ones most resistant to perturbation)
by which simulated systems can evolve increasing complexity of information
structure over time. This stability seems to be due to their optimising
geometric scaling of themselves and begins to confirm Phi-related
equations as imbedded within the study of classical dynamical systems -
and quite possibly within the larger body of quantum and relativistic physics
as well. This imbedding appears in a way that best transcends dissipation,
and minimises energy: hence emergent phenomena such as solitons, wavefunctions
and spacetime curvatures - and all that evolve from them.
So Phi-dynamics is a paragon of telos, it is an image of reflexiveness, of its own self-generative process translated in scale, over time. It is a conformally symmetrical recursive/invariant feedback loop acting as a rudimentary self-replicating memory machine (it reiterates its uniquely optimal relationship to, and between, Unity and itself - archetypal renormalisation), that therefore allows a manifestation in the linear and non-linear, at all scales, and in any higher dimensions. It therefore should also epitomise the principle of least action as its imperative, because this optimal stability should inevitably become the attractor for minimising entropy production/energy corruption: consequently, with Phi dynamical behaviour, information degradation is minimised, while system complexity is maximised. We can confirm these axioms by first noting that we can use the quadratic iterator to demonstrate not only the pervasive nature of Phi operation, but also its intrinsic relationship with dynamical systems. We already see that it is an archetypal feedback system that in turn generates a protofractal spiral that is literally linear (one dimensional), in form. This is seemingly paradoxical because to be fractal a form must be of non-integral dimension (between dimensions), in fact. However, the non-linearity is found in the scaling/rotation of the spiral, which is a logarithmic power-law (self-similar under transformation) behaviour. All fractals have this power-law invariance. Its dual linear and non-linear expansion of Unity is also paradoxical,
but the iterator:
Remarkably, even if we alter values for X[0] we still derive the same results. This makes the cycle as resistant to perturbation as is possible; 0 and -1 represent the repelling fixed points for the equation which in turn, generate a super-attractive orbit, between them. The orbit itself is strictly periodic but of the lowest dynamic period possible (being two) and therefore crucially, consumes the least energy to maintain. (Oscillation is also cheaper than rotation, and higher periods consume more energy.) This is whyPhi's deterministic switching action under infinite
iteration, must also have, following Boltzmann, a thermodynamic interpretation;
(after Roger Penrose) where:
So the constant c = -1 represents an island of perfect stability
surrounded by a seething maelstrom of Chaos. Solving the paired equations
for the system (known as a two-step loop) produced:
This therefore confirms Phi as the mathematical entity on the very cusp of Chaos. This is, considering its super-stability and attractive nature, a seemingly remarkable result --- which has been confirmed by several mathematicians and physicists in numerous and diverse fields of inquiry. (See bibliography). This point is of the highest mathematical significance for renovating our understanding of Phi in terms of dynamical systems and the immanent symmetry-breaking action of thermodynamics, from which they are derived. The Cantor Set is simply the equal division of a line into three parts, with the middle third being removed, scaling down to infinitely many intervals of finite length. It is the three-term division of One, as opposed to the two-term division of One by Phi. It underlies all Chaotic dynamics, in concert with Phi's switching action. This is because it has both a binary and ternary expansion --- and because it, too, communes between finite and infinite attractors. Chaotic systems behave in a kneading fashion, mixing-in infinite permutations: known as ergodic behaviour. This can again be described by a Ø binary operator. When we examine the Feigenbaum diagram of systems period-doubling into Chaos we find not only the Golden Mean to be a paradigm for all the bifurcations in it (a bifurcation is a Period Two event, and Phi is the locus of Period Two behaviour, see below) --- but also, that at the edge of Chaos, known as the Feigenbaum Point, the Phi operator produces an infinite Cantor Set, but crucially - with Phi proportions. The initial operations are illustrated (also see bibliography), in the Architectural Design Magazine (Academy Editions, London and New York) November 1995 article, which this review is a development upon . This Phi Cantor Set is a mathematical singularity (see above), analogous to an undecidable universal Turing machine. (A symbol-string abstraction that expresses dynamics as a form of infinite feedback of computational, reflexive information-processing.) Here in this zone on the edge of Chaos, entropy is minimal compared to the richness of information available; Ilya Prigogine described behaviours in this region as "the minimal entropy production (minimum energy loss), of far from equilibrium dissipative systems", and it can be seen as a typical example of the least action principle, in operation. Bands of order in the Feigenbaum diagram occur at a fixed scaling ratio, and correspond exactly to super-attractive periodic fixed points like those mentioned above. The bifurcations again, implicitly contain Phi. In fact, all the various universal constants found within the Feigenbaum diagram are derived from the Golden Mean's deterministic rescaling operation. This is how Phi is imbedded within dynamical systems, as a universal binary shift operator, or primary eigenfunction. All constants so derived are eigenvalues (think of resonances and harmonics) of this operator as it is constantly reiterated (as in nature), they are emergent (but not necessarily predictable) dynamic properties that often represent increased complexity, the further we move from the static, single-trajectory equilibrium state to the left of the diagram. The Feigenbaum diagram is also analogous to the symmetry-breaking behaviour of the early Universe as it lost energy and symmetry from it's initial unstable, highly symmetrical, far from equilibrium state. The critical difference is that such symmetry-breaking represented energy loss, whilst the Feigenbaum diagram describes the regaining of this lost energy - by returning to a more non-equilibrium, energetic and therefore, more symmetrical (and sensitive dependant, Chaotic) scenario. (The cosmologist father/son team of Andre and Dmitri Linde have actually proposed that the putative Chaotic initial condition of the Universe inevitably seeds an infinite, fractal Multiverse. (See bibliography.)) It is somewhat amusing to note that both Feigenbaum and Fibonacci (the originator of the series 0,1,1,2,3,5,8..., from which the Mean could be derived, nearly a millennium ago), are describing population dynamics, that of rabbits in the latter's case. The Rössler strange attractor (essentially an archetypal picture of a Chaotic system in four dimensional phase-space, like a section through a KAM torus) is used to model autocatalytic, far from equilibrium, self-organised sets such as the famous Belousov Zhabotinski chemical reactions seen at the Architectural Association's recent Complexity conference. Autocatalysis is the key to life's processes and it's initial and continuing evolution because it occurs on the edge of Chaos, where there is the greatest computational adaptability for the system, and where an emergent morphology can be best self-organised and then maintained over time, despite being immersed in constant environmental flux. The condition for such homeostasis being that for the reactions/entity to persist, reactants (potential or actual (low-entropy) energy/food) must be constantly added from that environment, and (high entropy) wastes returned to it - if it sounds familiar, it should: individual cellular organisms, their colonies, metabolisms in their multicellular cousins, groups of organisms, ecosystems, our entire human civilizations - and the planetary biosphere itself, display identical, scaled-up versions of this behaviour. Note the Phi spirals in the cellular automaton model. The attractor is a Cantor Set in 4D, over time --- and is another means of representing Phi in phase-space at the Feigenbaum Point, on the edge of Chaos. |

The Complex plane can be likened to the mapping of a sphere onto a plane and is the result of the curved Riemannian geometry used to model quantum and relativistic behaviour. A dimension shift is implicit here, as any triangle inscribed on a curved surface will have the sum of its internal angles add to more than 180 degrees. To move from one spatial dimension to another requires this type of operation. Theorised higher dimensions are believed to simplify physical laws to the extent that a unified theory of particles and forces linking the quantum and relativistic now seems within our grasp - they are the higher energy/symmetry, unstable dimensions referred to at the beginning of this review. Complex numbers have a real and imaginary component, so as to express planar co-ordinates, to higher, lower, (or between) dimensions - in a more complete way. (Imaginary numbers when squared, can still yield negatives.) If we then place two basins of attraction (imagine a pendulum and two magnets) in this plane we can perhaps simulate the behaviour of Phi at either the quantum or relativistic scales. We know the Golden Mean acts as a super-attractive orbit between two repelling fixed points, so if we again run iterations of the equation for the circle, with c = -1 (-1 being i, the imaginary number, squared), we produce a Julia Set, a fractal in the complex plane, (named after its originator), for the Golden Mean. The Mandelbrot Set is the encyclopaedia of all Julia Sets; it has been called the most complex geometric entity ever seen, is paradoxical in that it is a finite entity with an infinite boundary, and it too explicitly confirms Phi as of critical significance in its morphology. Again, with c = -1 we see Phi as the super-attractive origin of the Period Two Disk of the Mandelbrot Set. Geometrically, we are looking at sets with two basins of attraction: the infinite (ground) "escapee" set and the finite (figure) "prisoner" set. Iterating the equation yields one of these two results - points graphed to the complex plane land in either of these sets, to infinite scales and revealing infinitely changing details at their boundaries. Their boundaries are not only infinite, and infinitely complex--- but also contain self-similar, slightly mutated copies of the whole set. This boundary therefore, is the site of the instabilities between the two sets of figure and ground, which in turn yields new structures; it is the locus for the creativity of this set when viewed as either a static entity or as a dynamical system, and is an apt metaphor for the behaviour of those in nature itself. The Period Two Disk itself (centred on the Phi Julia set) acts as a geometric oscillator in dynamic equilibrium, between the positive feedback of the infinite escape set, and the negative feedback of the finite prisoner set. The Heart/Cardoid is Period One, representing equilibrium and stasis, meaning again that the lowest dynamic (time-evolving) period next to stasis is Period Two. The other Buds are all smaller, having higher periods, less probability and which again, consume higher energy to sustain. It is possible that quantum complementarity (particle/wave duality) and uncertainty (between position/momentum and energy/time) are artefacts of an analogous dynamic equilibrium (located on the boundary between the finite and infinite), that has been perturbed. For example, between the finite attractor of the period two evolution of the wave function of the probability amplitude for a particle, and the infinite attractor of the probability field it exists in, in terms of complementarity. For uncertainty, when you have infinite knowledge of a particle's position - you will have finite (zero) information about its momentum (incidentally, infinity is the mathematical reciprocal of zero). This is now known to be an inherent property of the quantum world, and not merely an observer-based artefact. Light behaves as either a particle or a wave, depending entirely on how we perturb (i.e.: observe) it. Perhaps the quantum world is only seen to be paradoxical and anomalous by us because we are constantly seeing it being perturbed from an ideal ground (minimum energy) state by its inherent fluctuations; for every metastable state, there always appears to be a yet more stable scenario, hence phenomena such as quantum tunnelling to lower energy states (as the least action principle would dictate). String and Membrane theory are based on the least action principle because of its elegance and beauty, and express both matter particles (fermions) and energy/force particles (bosons) in higher dimensions (and at cosmological initial-condition energies - reproduced at their 10 to the minus 33 cm Planck scale/energy size, because of Heisenberg uncertainty at this smallest possible scale), as rotations, resonances and harmonics of vibrating entities that are both energy/matter and space/time. The quantum and the relativistic are therefore united by these entities; whose harmonics are now seen to be directly analogous to solitons (persistant travelling waves), which at classical scales are only found on the edge of Chaos. The implication here, is that scaling up from Planck scales, the quantum collapse of the wave function is analogous, by scaling, to classical irreversible non-linearities, as both require the disruption of a system from a dynamical equilibrium, thereby increasing their entropies. Wavefunction collapse therefore may in a certain sense, be a quantum precursor of the classical-scale bifurcation, leading eventually to the edge of Chaos. This may be why trying to find full Chaos at the quantum scale is proving fruitless, the causal direction of non-linearity probably needs to be reversed: String/Membrane soliton harmonics leads, to quantum uncertainty/complementarity, which in turn, leads to classical instabilities and Chaos, (and therefore the creation of emergent, self-organising information structures of mass/energy on its edge), not the other way round. Each increase in scale sees emergent patterns of mass/energy, that although outwardly unrelated, display the same underlying metapattern - which is because an increase of scale is also evidently, an energy minimising, symmetry-breaking, dimension-compactifying process. If we look closely at the Phi Julia Set, we can measure 1:1/Ø**2 in its proportions; exactly Phi's reciprocal, quadratic action. The Complex plane itself can be mapped by a system analogous to electromagnetic/gravitational force equipotentials and field lines. When we describe the Phi Julia Set fixed point behaviour, we see two field lines landing on the pinch-point (as with the cones/spindle example) for 1/Ø, representing an angle doubling from 1/3 to 2/3 of 2pi. Now, angle doubling in the Complex plane is equivalent to squaring in the Euclidean plane, and to an oscillation of the binary shift operator. This action should therefore confirm Phi's behaviour, as an operator, to be applicable to the quantum and relativistic (linear and non-linear) domains as well as within dynamical systems, at the so-called classical scale. (Note that space-time itself is fractal: curved and non-linear. For example, the inverse-square law of gravitational force versus distance is a simple fractal, and therefore self-similar, power law. Gravity is space-time curvature - caused by mass/energy - its manifolds are always traversed in minimum-entropy geodesics, lines of least distance; again, just as water flows through the lowest points in a valley, thereby minimising its gravitational potential energy.). Returning to the Mandelbrot Phi Julia Set itself, we see that the fixed points are on the boundary between the finite prisoner and infinite escape sets, on the edge of Chaos, in fact. The Golden Mean is therefore intrinsically dynamic, its action is one of perpetual reciprocal oscillation, exactly and uniquely replicating the dynamics of the unit circle. The Phi Julia Set, centred at the origin of Period Two dynamics, is the only set that acts so.
This again, means Phi behaviour seems to operate at all scales and in any dimension, and confirms it as when infinitely iterated, the mathematically most stable attractive orbit for achieving a mathematical singularity. The fixed points themselves confirm the orbit between the finite (0) and infinite (-1) attractors. The finite attractor here is the period two orbit, analogous to a graph of: y = sin x. The infinite attractor is that same orbit as the graph of the reciprocal: y=sin(1/x). Remarkably, these graphs of simple harmonic motion can be used to describe the curvature of space-time in approaching the speed of light (relative to an outside observer, i.e.: the rest of the Universe, time stops, you compress to finite size, as one dimension compactifies, and gain infinite mass - unless you are initially massless, like a photon), or the approach to (or departure from), a space-time singularity such as a Black Hole, or the Big Bang (when times also stop) - which are intriguingly and non-coincidentally, time reversals of each other. The squaring of the circle therefore, has an expression in the Complex plane, which confirms the Golden Mean's profound dynamical and symmetric action, and potential mathematical application to quantum (linear) and relativistic (non-linear) physics, the two limits below and above, the classical (which combines both behaviours). Of course, the very small and the very big, the quantum and the relativistic, are as stated above, believed to be linked by String/Membrane theory: together producing a unified formulation of quantum gravity. This theory is essentially geometric (specifically topological), always dealing with minimal surfaces, albeit in ten, five dimensions for String and eleven dimensions for Membranes, and are predicated on energy-minimising processes. The major outstanding issue in String/Membrane theory is how the dimensions compactify (since we only experience four - three spatial and one temporal): how they break symmetry and bifurcate, minimising their energy to produce the stable masses of the elementary particles and the physical constants within our four dimensional space-time. To speculate, one could hazard that the edge of Chaos (also known as self-organised criticality) might be the actual global, dynamical process of the Universe itself with phenomenological examples or empirical proofs possibly being in evidence of criticality in static states: such as finding Phi proportions in the vascular distribution, structural organisation, and space compactifying of organisms (which we do), the root-five symmetries of the DNA molecule (ditto), or other physical permutations of Fibonnaci numbers and their complements, the Lucas numbers, or self-organised critical, power-law behaviour - in dynamic processes. Remarkably, Fibonacci numbers do crop up in various analyses of the rest-mass (minimum energy) relationships of the elementary particles - but also (scaling up again), in solar system orbital relationships (such as the main gap in the rings of Saturn); or self-organised criticality is revealed by the soliton on Jupiter, better known as the Great Red Spot, or by the Sun's dynamical behaviour, or by the x-ray emission spectrographs of Black Holes devouring matter from their accretion disks. Perhaps the non-locality, the global features of the edge of Chaos region, hint at an answer involving quantum time-reversibility for the famous EPR paradox - relating to elementary particles appearing to be in touch with each other without any time delay and over apparently cosmological distances? Perhaps we now have a good reason why. Perhaps we are looking fundamentally, at various harmonics and resonances of the string/membranes (as with KAM tori), that display the most self-organised stability for the least energy, at criticality, the edge of Chaos. The complex relationships between all the (nested) tori due to the broken symmetries of our energy-minimising, non equilibrium Universe yield the various resonances, harmonics, (and constants?) of our world, whether we are discussing Planck, Plate Tectonic, or Parsec scales. These same rules also seem to apply to Protoplasm, Punctuated equilibria, Politics and the Price mechanism (see Mandelbrot on cotton prices) - and are therefore about as universal (and as relevant to cultural production such as architecture), as they could get. Perhaps our wan ember of a Universe has evolved, has self-organised itself to a critical state on the cusp of a Chaotic, infinite possibility space via its symmetry-breaking, energy minimising, unfolding journey from the highly energetic, low entropy, supersymmetrical - but chaotically unstable conflagration of the Big Bang? If we then compare the Mandelbrot Set with the Feigenbaum Diagram we can see how the Period One Cardoid of the former corresponds exactly with the single, equilibrium trajectory of the latter. The Period Two Disk with Phi as its super-attractive centre, exactly matches the first bifurcation --- confirming Phi, geometrically as the paradigm for deterministic bifurcation. All the subsequent bands of order in the latter (which occur at a fixed scaling ratio derived from Phi-action), precisely match the micro Mandelbrot Sets along the the horizontal, real-number axis of the Set. This geometric evidence defines Phi then, as the optimum oscillating operator that mediates between ordered, equilibrium systems and disordered, non-equilibrium ones. It allows an oscillating orbit to access the infinitely fecund chance morphologies of Chaos, recapitulate them back into its super-stable causal orbit: and therefore permit system growth and morphogenesis. It is the paradigm for systems evolution: for the emergence of global, causal complexities from local chance simplicities - for bottom-up evolution based on hierarchies of increasing sophistication, themselves engaged in continual feedback, catastrophes and transient stability's. For Phi behaviour then, chance and causality, Chaos and Cosmos (the Greek for order), are in the tension of a dynamical equilibrium; an equilibrium that creates the unexpected and the emergent, which with each symmetry-broken hierarchy, yields transient morphologies of often increased complexity. The Golden Mean therefore represents absolute global deterministic order, as an iterated period-doubling (bifurcating/symmetry-breaking) system, but over time, also allows for local, stochastic Chaos, chaos which can, when the system is far enough from equilibrium ( as a fractal, self-similar "ghost" copy of the initial condition - the very non equilibrium Big Bang), also create. Since the passage from one to the other is governed by the second law of thermodynamics, responsible for irreversible processes (beyond the reversible and linear quantum world), this also makes Phi behaviour the optimum operator for both linear reversibility and non-linear irreversibility. For a system to reach and maintain maximum complexity as entropy increases, (something which is progressively harder when scaling up from the linear, reversible quantum world), the optimal way to avoid ever-increasing resonances (interference) and instabilities, is to preserve a Mean minimum-entropy trajectory. The Golden Mean in effect, allows an infinite fractal (the Cantor Set mentioned earlier) to tap the creativity of Chaos, for the use of order, an order which is entirely for free, (except for entropy-tax!), making us both part of an implicate unfolding - as well as the result of an explicate vicissitude of the cosmological casino. This transaction occurs where the algorithmic complexity of a system is maximised, on the edge of Chaos; so, if maximum Complexity is (to use a surfing analogy), the board which surfs the wave of inexorable entropy increase, then the Mean is the (least-action) optimum, most elegant and energy-minimising path along the face of that wave. Various cellular automata ( heuristic pixel matrix) simulations also may confirm Phi's system structure-enhancing evolutionary action, to the point of researchers seeing this binary-switching behaviour as an inevitable target for natural selection. Stuart Kaufmann, biologist and computer scientist at the Santa Fé Institute, in promoting his view that Complexity explains evolution as a relationship between selection and self-organisation; and the order in the genome and the emergence of life itself; cites binary arrays displaying apparent period two behaviour as those allowing "broad boulevards of possibilities - rather than back alleys of thermodynamic improbability." Kaufmann's CA's place the regions of maximum complexity, islands of stable pixels surrounded by chaotic switching ones ("frozen cores" in his terminology), firmly in the self-organised critical/edge region. This behaviour, used to (accurately) predict genotypes for organisms of different complexity, results when his arrays are linked together in which each pixel responds to the input from two others, but no more or less - (less produces stasis, more increasing chaos).This period two-analogous behaviour, in what he calls his Boolean NK nets, shows Phi in action yet again: this time, in silica - as well as in the vivo of reality. His work extends to whole ecosystems, and includes the realms of fitness landscapes and simulated annealing, where robust, energy-minimising (fitness enhancing) strategies are all revealing deep links between ecology and economics. The same can be said for various investigations into plasma and quantum-scattering physics, pure maths of dynamical systems, fractal dimension, and ecosystem simulations --- to name a few. All cite Phi-behaviour as an invariant attractor behaving in some or all of the ways described above. (Please consult the bibliography references.) The physicist Per Bak models Self-Organised Criticality, a term he coined: in these simulations he observes reciprocal squaring, power-law behaviour. This law is ubiquitous in nature, whether it is sand-pile avalanches, earthquake intensity over frequency, neurone firing patterns during consciousness, stock market behaviour, or the previously mentioned celestial, Black Hole pyrotechnics. (This is the remarkable feature of Complexity incidentally, that the hitherto unrelated: the inert and animate, natural and human-metabolic or cultural phenomena, all seem subject to the emerging new paradigm of criticality on the edge - all are linked in a new universal rubric.) This behaviour is also self-similar at all scales, and is reputed to display energy-minimising behaviour as it gravitates towards edge of Chaos criticality, and so it seems reasonable to intuit it to be another manifestation of Golden Mean dynamical behaviour. Chris Langton, also of the Santa Fé Institute, dedicated exclusively to Complexity research, likens order to a solid (like ice), disorder to a gas (steam), and believes a liquid (water) form of Complexity occupies a phase transition region between the two. The Institute is essentially exploring this region first popularised as the edge of Chaos by Langton, by means of various computer simulations, looking for a putative new formulation of the second law of thermodynamics, (a search this review is commenting upon, but also hopefully contributing to!). What the Institute finds is that the liquid/phase transition region behaviours are the most complex, the most stable under perturbation, display non-local (global) behaviours, and are capable of universal computation - with extended, transient entities emerging spontaneously from them that inevitably, because of their qualities, have been dubbed "artificial life". Since life is the most complex known feedback-led process in the Universe, and consciousness the apotheosis of these processes: the simulations of artificial life could be extremely promising in promoting our understanding of how such global complexities can emerge from local simplicities. Once again, non-locality is a proven riddle of the quantum world (paired particles behave as if in instantaneous communication - a violation of the speed limit of light); and additionally, the phase transition is integral to inflationary theories of the symmetry-breaking expansion of the early Universe. Langton cites the phase transition zone as that containing the most potential complexity for the entropy generated. This is because the cellular automata used then behave as undecidable universal Turing machines, as universal computational singularities, exactly replicating the Feigenbaum Point dynamics referred to above. We then are in the number theory realms of the NP problem and Chaitin's Omega. Considering the evidence above, it is likely that the Golden Mean will form a significant part of these explorations. This is because the Mean appears to be just that: an optimum oscillator in dynamic equilibrium between entropy increase (information transmission), and entropy decrease (information storage), thereby sustaining maximum complexity in a computation, over time. In terms of coin-tossing behaviour (50/50 stochastic binary-switching), Omega appears to be analogous to Mean dynamics at the Feigenbaum point: and potential further evidence that for this case, NP-complete problems are intractable, universal Turing machine halting is undecidable, algorithmic complexity is non-computable, and of course, that this situation conforms to Gödel's incompleteness theorem. Number theory therefore also confirms the Golden Mean to be on the very cusp of Chaos, but also the quantum, via Reimann's zeta function, in quantum scattering.. Essentially, all these results point to the inherent limitation that to be self-consistent, mathematics must admit the random - that not all unproved statements are provably unprovable ("All Cretans are liars", spoken by Epiminedes, a Cretan). Sometimes a statement is too complex and reflexive to be proven - one falls prey to tautology and paradox. Nature is subtle - but not malicious, to paraphrase Einstein. (Who told Corbusier that his Modular, based on the Mean, "made a difficult job more easy"!) Causality must admit, dance with, court, marry and (pro)create with chance. Local chance events underlie and create our apparent reality in the most fundamental of ways, and yet they paradoxically are the basis for the global causality we see all about us. For Prigogine, the true nature of reality, the new paradigm - is the best of both worlds: somewhere in dynamic equilibrium between. Pure Newtonian causality is an incorrect (finite) view, but then again, so is the aspect of complete uncertainty and (infinite) chance: our world is a dynamic synthesis of the two. The edge of Chaos region is therefore the most globally stable (remember, the cellular automata reveal structures known as extended transients in this phase-transition zone), but it is also the most locally creative. Phi resides in this optimum boundary zone: between the causality of the bifurcation branches, and the chance of the bifurcation points themselves: between the result of the coin-toss and the initial toss itself. In terms of Phi-Julia Set behaviour, its super-attractive (global) orbit is optimally stable because it is mediating between those two optimally unstable (local) repelling fixed points. This local energy-minimising behaviour, when applied to the arbitrarily large numbers of shift-operations implicit within say, the complex neural and metabolic processes of individual organisms - allows global stability to be maintained in the face of an environment in constant flux. This stability has just enough creativity in it to deal adaptively with new situations. This might be on the scale of an individual organism such as an Echidna, or its staple prey, an ant colony. A flux between order and randomness, with not too much of either extreme, is the key to any act of both evolutionary adaptivity and/or homeostasis. Consciousness itself might well be a form of optimal, adaptive information processing, a learning, fluid homeostasis of neurone-firing, one which has been theorised in the Institute's journal, titled "Artificial Life", (and endorsed by Roger Penrose), to be further based on the quantum switching of single electrons on dimer "switches" located like CA pixels on microtubules, filamentous structures that are purported to be the nervous systems of individual cells. Such CA simulations display edge of Chaos extended transient, glider and other complex (specifically, Wolfram: Type Four quasi-periodic/Conway: Game of Life) behaviour typical of the other cellular automata simulations listed above. It seems clear now that the Golden Mean can certainly be reconciled with the new science, which reveals a profound dynamical aspect to its action. The Mean can be found to mathematically describe the behaviour of nature in at least three major idealised models. Firstly, the final Phi-stability of quasi-periodic KAM tori, just prior to collapse into chaos. Secondly, the universal behaviours, Phi-Cantor set at the Feigenbaum point and constant scaling relationships of the period-doubling Feigenbaum diagram. and Thirdly, the most complex, liquid/phase transition region of cellular automata behaviours, displaying quasiperiodic, period two and therefore, Phi extended-transient structures. All locate the Mean as the route to, and on the edge of, Chaos, in the realm of maximum complexity - as exchanged for minimal energy waste, or entropy production. The Mandelbrot Set is the geometric icon that links all three in a lapidary, metaphorical, almost mandala-like sense, but that is itself, also generated by equations that tell the same story. Number theory is saying that this is the ultimate, deep message contained in all self-consistent logical structures, in the relationship of relationships (as mathematics has also been poetically called), causality and chance - dance on the cusp, creating the increasingly complex in their wake. So we now also know that causality and chance, Cosmos and Chaos
if you prefer, do not waste precious energy/mass (it is minimised), symmetry
(it is broken), dimensions (three spatial dimensions are the liquid/complex
region in random walks: less, and not much interesting happens - more,
and you get hopelessly lost), nor time (it too, breaks directional symmetry
above the wave-function collapse (lowering its energy) - and is linked
with the three spatial dimensions in geodesic curves that express energy/mass
as the weakest known force - gravity) .
Without prolonging this review excessively, here are some brief examples of how the assimilation of this new knowledge of dynamical systems, married with new environmental and structural engineering, plus materials and information technologies, might reinvent the statics of architecture (without simply resorting entirely to static proportional applications of the Golden Mean, which are always distorted by the fractal scaling of perspective anyway, or to pastiching past aesthetics): The first emergent feedback-led application is in the design process itself; the form(al cause) of a building being the result of the consultative process and the cultural milieu that informed it. If this process fully involves the user and all other concerned parties, and takes full note of all the brief and site constraints and histories; a democracy of rich mutual feedback will result. The problem papillon of sensitive dependence will hopefully sleep. The architect can then act as mediator, co-ordinator and (auto)catalyst for the emergent, complex form that arises, which appropriately should epitomise the "final cause" of least action in being the optimum artefact for its role, (and also by being energy-efficient, where possible). Speaking metaphorically, one could say that the architect in this light, almost personifies the optimising imperative of Phi - as generator of information, in their role in the design and production process. Climate control will increasingly become a hybrid of feedback between active elements, such as mechanical HVAC, and the passive, such as natural ventilation. Low-tech, vernacular-inspired strategies for climate control, such as the atrium, fused with the higher technology of computerised brise soliel solar-gain louvres with integrated photovoltaics, for example, are already in use. The future is dynamic, adaptive green building. Stack-effect, evaporative cooling, cross ventilation, solar chimneys, wind towers, buffer zones in the multiple skins of building facades, plant biomass evapo-transpiration (also enhancing homeostasis of oxygen/CO2, expressing seasonal variations - and their own fractal structures), and even aerodynamics - are some of the hybrid strategies currently in use. (Note here that the Lorenz attractor, related to the Rössler attractor, is a model of thermal convection flows - and therefore directly applicable to passive climate control design on a micro-climatic scale.) All are systems (or functions) leading a potential emergent form. A facade incorporating electrochromic glass that is linked into
a CPU hosting a parallel-processing neural network can instantaneously
regulate solar gain by adjusting facade opaqueness, depending on it's orientation.
That network can also minimise overall building energy load by only lighting,
ventilating, heating or cooling occupied spaces. Such systems may display
a form of metabolic homeostasis known in artificial intelligence circles
as again, emergence; allowing for simple system-memory and learning capacities.
This has everything to do with emergent, recursive feedback loops being
employed in environmental service design. Clearly, dynamics can synthesise
with statics. The relevant question is one of the appropriate level of
automation, of the dynamics, the type, and how they impinge on the static
form of the building. Intelligent agents imbedded in the electronics of a buiding and
its contents, will become another ambience that fills inhabited work and
living space with continual feedback, making the experience of that space
become a form of customised, cybernetic extension of the consciousness
of the users.
A major design constraint (and therefore, opportunity) for passive
climate control is that strategies and final design solutions must adapt
to the local climates (and therefore inevitably, the cultural readings)
of sites: hence the earlier use of the term "Vernacular", as a methodology,
(but not an aesthetic of pastiche), of Complexity, as applied to an autocatalytic
architecture, that conveys thematic and structural analogy (self-similarity,
or reflecting this in society as a whole), and thermodynamic (historical)
depth.
However, vernacular buildings saved energy (and were generally
not profligate with materials nor structure), so as to create a sustainable
living and working environment. They were appropriate, creating a symbolic
rubric of available materials and forms connoting a cultural response to
a particular environment. The vernacular was emergent, bottom-up,
distributed and parallel, hence its veracity. It conveyed an irreducible
order: making the whole greater than the sum of the parts, a gestalt in
effect. A combination of the bottom-up and the top-down,( the imposed solution
based on the personal visions of client, planners and architect), would
therefore, seem the right combination for most contemporary urban environments.
Any architecture that minimises local (and therefore global) entropy-production
(information and energy waste), by fusing passive/active strategies to
optimise energy load; in exchange for optimised emergent complexity (or
contradiction!), be it fractal detail for the eye at different scales,
or minimum structure for maximum strength - or opposing tensile with compressive
elements, the dynamic against the static; will be more likely to succeed
in its purpose. It will form a better cultural response, a more apt metaphor
for our understanding of reality; surely the ultimate goal of all art and
science - (both of) which architecture should ideally, epitomise.
It must be stressed that this is not an appeal for the strict
imitation of the vernacular but rather a recognition that just as in the
past, differering climates and cultures should naturally produce different
design solutions, and produce distinctive, discrete "bioclimatic" architecture
appropriate to their climatic and cultural environment. This produces a
distinct, profound regional identity and variety for architectural
form and material throughout the world, as we are already seeing.
The Modern Movement's main failure was that it became a metaphor
for the hubris of the Newtonian mechanistic paradigm. It imposed an anonymous,
excessively top-down homogeneity worldwide (the International Style is
also the most internationally loathed). It ignored global vernacular architectural
diversity of cultural response; to a plethora of environmental constraints
--- and so identity was forbidden for the Machine Age. How then do we
transcend this failure --- and restore identification (which is a form
of empathic self-similarity)? What this investigation of the dynamics
of the Golden Mean tells us is that it is an analogical, optimum mathematical
paragon of energy efficiency: and that this is achieved as an ultimate
means of generating the maximum complexity of information structures of
energy and matter, at the lowest energy cost. Rather than merely imitating
the statics of proportions, we are now also liberated to epitomise the
Mean through elegance of: structure (the bearing of dynamic forces), efficiency
of organisation, gestalt, contrast, congruity thematic/contextual resonance,
reflexiveness, irony - and the economies of
vernacular-informed green
architecture.
This is the methodological genius loci of Complexity; of emergence,
of celebrating diversity and contextual veracity over homogenised anomie,
of the rational pursuit of the irrational, of applying engineering elegance
and efficiency as the proper syntheses of the dynamics of function with
the statics of form. The role of architect is to catalyse the emergent
form of a building or a website (or any virtual entity) from a possibility-space
that yields the optimal complexity (or algorithmic depth, if you like),
required for that particular project. Because the constraints generating
that complexity can be wildly divergent (just like symmetry-breaking and
instabilities far from equilibrium), this can be a challenge, to say the
least, analogous to that of reconciling art with science.
Architects have to relate the the most divergent of constraints,
from applied science and physics/ engineering to conceptual art, from mathematics
to colour theory and psychology; from adminstration to surveying to law,
from projective geometry and computer science to graphic design, to name
a few. This also makes producing truly great architecture one of the hardest
tasks in the world. The fact is, the accuracy and iterative power of computers
have allowed us to reveal the true, vastly rich temporal structure of dynamical
systems - making this site possible, but they have also brought the two
cultures of art and science closer together - back towards perhaps, Renaissance
(if not Pythagorean!) levels, where both again use the same language of
geometry and the conceptual. Both now construct simulations and simulacra,
both map nets of knowledge, and create models, or archetypes of reality.
Intellectually, it is therefore perhaps fertile ground again for architects:
for the practitioners of projected spatio/temporal geometries, to also
engage in the semiotics of geometry and concept, in terms of that reconciliation
of the two cultures.
Complexity makes this more possible because less is lost in translation:
the two languages (of the two cultures), are being synthesised, through
an increasingly shared use of the commom languages of geometry and analogy.
Complexity synthesises the sciences, be they physics, chemistry, biology,
ecology or economics, history, politics or cultural studies, physical or
life science, "hard" or "soft." It does this by using mathematical models
(with parameters partially based on intuition), as a codified geometry
- so simulating and testing archetypal models of natural systems rather
than
actually experimenting on the systems themselves (because of their high
complexity). This has brought science far closer to the intuitive, conceptual
and visual language of art than ever before in modern history. So architects
can (at least intellectually), find that less will be lost in their translations
between the two cultures.
A work of art is deemed beautiful if it possesses gestalt, is
seen as more than the sum of its parts, if it is conceptually analogous
and/or physically fractal.
Science tells us the world is poised in an analogical self-organised
critical state, with fractal spatio-temporal structure. This knowledge
system itself has the same structure.
Both conditions are found in the basin of attraction at Chaos's cusp.
A functioning, built work of architecture can also be seen as
a dissipative, open system just like an organism: which has a limited lifespan,
which consumes (for example), low entropy electricity to produce high entropy
heat, as an act of metabolism. The less entopy produced, the less energy
wasted. This can be achieved by an apt mix of technologies and materials
(low or high tech/spec), for the local benefit of the immediate users,
but also for that of the global environment. Since the energy servicing
of buildings (especially heating, cooling, ventilation and lighting), produces
50 per cent of all greenhouse gases - these issues are not trivial, and
will with time, become unfortunately, even less so.
The sciences of complex dynamical systems show how appropriate
it is to perceive our age as one of information: one which increasingly
exists to exchange and enhance pure information to higher levels of complexity
at minimal expense (recall the global internet itself, priced at local
phone rates), just as the irreversibility-driven evolution imperative of
the Universe and of life itself demonstrates. Architecture, as a cultural
artefact that inevitably will be imbued with the current zeitgeist, should
assimilate as much of the implications of Phi/Chaos dynamical symmetry;
as its procurers, practitioners and pedagogues will allow. We have the
opportunity to (re)present the Golden Mean to architecture and society
in entirely novel ways, beyond Pythagorean pentagrams and pentagons, geometric
analyses of the Great Pyramid or the Parthenon, or Euclid and his geometries,
or Fibonacci, Alberti and his Florentine churches, or Piero della Francesca
or Kepler and Platonic planetary orbital ratios, Cook and plant phylotaxis,
or Hambridge and his dynamic symmetry, Le Corbusier and his scheme to replace
both imperial and metric systems with his Modular, Cartier-Bresson with
his "decisive moments"and classical composition of his reportage photographs;
beyond statics and state, but rather via dynamics and process (as Cartier-Bresson
intuits): as tension, as paradox, as a dynamical unity of opposites (finite
and infinite), fusing the linear with the non-linear, the ordered with
the random - and causality with chance, to create the emergent and unexpected.
This is the (ana)Logos of Heraclitis of Ephesus, or the
Tao
of Lao Tsu. These concepts seem to be part of a universal global archetype,
in the Jungian sense, to have been already intuited separately by other
cultures, at earlier times.
More pertinently, James Lovelock's Gaia hypothesis, (where the
biosphere of the planet itself is self-organised), is a paradigm for a
truly global homeostasis based on Complexity, where the whole planet is
posited to minimise its entropy-production at the edge of Chaos,
(fixing gas proportions at non equilibrium levels suitable for life, for
example), because of the cellular automata-like action of the biosphere.
We have a duty to future generations to learn from Gaia-esque
concepts; to preserve humanity, its cultures, and the global ecosystem
(and their respective diversities), to live by the maxim of sustainability,
which is to: "not make the world a worse place for our children by our
current actions or inactions". We have a moral responsibilty of course,
but also one based on preserving the complexity and diversity of the only
known seat of intelligent life in this Universe. We and our world are like
the Mandelbrot Set: the most complex and diverse known entities in existence.
The Golden Mean, because of its supreme economy, elegance and capacity
for analogy, because it resides where it does in dynamical systems behaviours
such as within the biosphere, is therefore also the ultimate metaphor
for sustainability. Etymologically speaking, the architect is a "fabricator
of archetypes"; these ideas and issues as discussed above are as profound
as they are pressing. If we are to mitigate our worst ecological excesses
(in better understanding and replicating nature, we can better preserve
it), or to create better examples of criticality: metaphors and analogies
of reality (with these archetypes), we must assimilate the lessons of the
sciences of Complexity, for they are the laws and emergent properties of
nature itself.
Suffice to say, maximum Complexity is found via self-organised
criticality at the edge of Chaos, which is epitomised by the Golden Mean,
as the emergent geometric manifestation of the principle of least action:
therefore its full temporal/ spatial action is analogous to creation itself.
|