Pentomino Fuzion Challenges

6x10 solution The rectangular 6X10 board has probably been studied the most. There are exactly 2339 different solutions but it is surprisingly difficult to find one. As a variation try finding a solution where all 12 pentominoes touch the outside perimeter. Another challenge is to find the maximum number of pentominoes that can be placed in the interior without touching the perimeter. The solution shown here has 3. There are 32 interior squares which would allow 6 pieces, but clearly there are not enough long skinny pieces to go single file around the perimeter. Can you find a solution with 4 or more pieces surrounded?

5x12 solution The rectangular 5X12 board has exactly 1010 different solutions. When using computer programs like Fuzion, duplicate solutions can be eliminated by placing the X pentomino in the same quadrant every time. The X piece is chosen because it has only one possible orientation. That is, it fits the same in a puzzle when rotated and flipped-over. Which pentomino has only two orientations? There are six pentominoes that fit differently for each of the eight possible orientations, can you find them?

4x15 solution The rectangular 4X15 board has exactly 368 different solutions. The solution shown has 2 interior rectangles, so 16 different solutions can be easily made by flipping through box combinations. The 3X20 rectangular board has only 2 related solutions. The solution isn't shown so you won't be tempted while working on this challenge. Some people feel the 3X20 solution is easier to find than one of the 6X10s, contrary to the tale in Arthur Clarke's Imperial Earth.

Triplication Z Imagine magnifying a pentomino so each square is divided into a 3X3 grid of 9 equal squares. Do this to each of the 5 pentomino squares to yield 45 smaller squares. It is now possible to fill the shape with 9 of the 12 pentominoes as shown here. Can you find a solution to each of the 12 pentomino shapes? Take a peek at all 12 solutions. Which pentomino shape has the most solutions? Which has the fewest? Fuzion's board designer and solver comes in real handy here.

8x8 solution A checkers board has 64 squares so if 4 squares are disallowed, pentominoes should fit around the "holes" on the remaining 60 squares. The challenge is to place holes making a board that can not be solved. Excluded are the obvious methods of isolating board squares. For example placing 4 holes around in a circle with only the corners touching or 3 holes touching corners along the perimeter. In other words it must be possible to place at least one of the pentominoes in the areas around the holes. Here is a page full of checkers board solutions. To date there are no known unsolvable 4-hole checker boards.

double stack The challenge is to find two identical sections as shown here. When placed on top of each other they overlap exactly. Each half section will contain 6 of the 12 pentominoes. How many different shapes can you find that duplicate? It is well known that two 5X6 rectangular shapes can be solved. One visitor to this site discovered a method of assembling 3 equal sets of pairs (solution spoiler) which can be combined to create many duplicates. Can you find any other 3 equal sets of pairs?

family of 3 Try to find a family of shapes as shown here. It is easier to see then explain the rules. The big shape in the middle is twice the size of the two smaller shapes. Start by finding a shape with 2 pentominoes, then see if you can duplicate it with 2 other pentominoes, finally use the remaining 8 pentominoes to form the double size shape. To date visitors to this site have found 3 other sets meeting this criteria.

3 = sets The solution shown here is a family of three congruent shapes and was given in Golomb's new Polyominoes book. These solutions are a fun way to store 3D pentominoes in a compact stack. Fuzion's board designer and auto solver has found several more solutions. Many other probable boards have been ruled-out as unsolvable by Fuzion. Can you find any more solutions?

Solutions Library

To get involved in the ever expanding knowledge of polyomino possibilities you can send Email to the Fuzion Database. If you are playing with Fuzion simply "attach" a copy of the pertinent [].SOL file to your email. The [].SOL files are located in your Fuzion directory. Each board has a separate solutions file for example the rectangular 6X10.SOL or the 8X15.SOL file for puzzles made with the board designer. If you are playing manually you could represent each pentomino with sets of different letters as shown below.

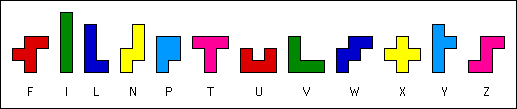
If you have a new challenge, questions, or comments we would be glad to hear from you.

View basic Polyomino statistics

Send Email to the Fuzion Database

Download the latest shareware version 2.8.JAN2000 of Fuzion! (95 kb)

[ FUZION HOME ] [ CHALLENGES TOP ] [ LINKS ]

Click on Fuzion Home to transfer to the most current Fuzion site.

This page hosted by

Get your own Free Home Page