 times.
times.
This location was visited  times.
times.
![]()
1. In Session 81 HW Question 2: what if dimensions of a table to be covered with dominoes were 100 x 100? 100 x 101?
2. Find square roots of a) i; b) 5+12i
0. Find square roots of 8+6i.
1. A square with dimensions 4 x 4 was cut out from 8 x 8 chessboard and it is known that the rest of the board can be dissected into 1 x 3 triminoes. What was the position of the square inside the chessboard?
2. One hundred businessmen attending a conference occupy 100 chairs forming 10 x 10 square. Each of the participants inquires of all of his/her neighbors (in front of him/her, behind him/her, to the right and left of him/her, and diagonally) about their income and if no more than one of them makes more money than he/she we will call such a person "satisfied". What is the maximum possible number of satisfied people attending the conference?
3. Find six points on the plane such that any five of them can be covered by two squares with unit diagonals but all six of them cannot be covered by two circles with unit diameters.
4. How many solutions are there for the equation 19[x]+97{x} = 1997 where [] and {} stand for integer part and fractional part functions (e.g., [3.14] = 3, {3.14} = 0.14).
![]()
1. Does the first quadrant of the complex plane represent a group with respect to addition? to multiplication?
2. Do the set of complex numbers with integer coordinates and operation of addition constitute a group? If the operation is changed to multiplication? What if integers are changed to rational numbers?
3. Find an inverse to z = a+bi, that is, complex number t such that zt = 1.
1. Two equilateral triangles ABC and PQR (vertices listed clockwise) are positioned on the plane in such a way that point C lies on PQ and point R belongs to AB. Prove that BP is parallel to AQ.
2. Eight volumes of selected works by Jules Verne are standing in a row on a shelf in soke order. It is allowed to take out a volume that occupies either the third or the eight place (from the left) and place it in the leftmost position. Prove that using such operations you can always achieve the correct arrangement of the volumes.
3. Prove that you can delete one of the one hundred operands in 1! x 2! x 3! x ... x 100! so that the product will become a perfect square.
4. Forty-nine backlit buttons are arranged in a 7 x7 board. Pressing any button changes its state as well as the state of its neighbors (including diagonally positioned): light to dark and vice versa. Prove that by pressing buttons one can always arrive to the completely dark board (starting with any initial state).
![]()
1. How many complex roots does a quadratic polynomial with complex coefficients have?
2. Prove that any permutation can be represented as a product of transpositions (i, i+1).
1. Eight rooks are positioned on a chessboard so that none of them is under attack by any other. Prove that the number of the rooks in the upper right quarter of the board is equal to the number of the rooks in the lowert left quarter.
2. In triangle ABC angle bisector of angle A, altitude BH and perpendicular bisector for side AB meet at the same point. Find the value of angle A.
3. Find all natural numbers n such that the sum of the squares of all their proper (not equal to n) natural divisors equals 2n+2.
4. On the island of knights and knaves in company Knick-Knack
Inc. one day each one of its employees made the following two
statements:
a) less than 10 people in this company have greater
workload than I do;
b) at least 100 other employees have salary
greater than mine.
How many employees could be there in the
company (it goes without saying that all the salaries and the
workloads are different)?
![]()
1. Prove that for any natural number n that has more than four natural divisors the product of its greatest two proper divisors is greater than n.
2. Prove that the product of two polynomials is a polynomial and find a formula for its coefficients.
3. Prove that the degree of the product of two polynomials equals the sum of their degrees.
1. A rectangle ABCD is dissected into squares as shown on the picture. It is known that AB equals 32 cm. Find the length of side AD.
D _______________________________ C
| | |
| | |
| | |
| | |
| | |
| | |
| | |
|______________| |
| | | | |
| | |__|_______________|
| | | | |
|_____|_____| | |
| | | |
| | | |
| | | |
| | | |
A |___________|_________|________| B
2. All the numbers from 1 thru 10000 were written in ascending order on a blackboard and then all of them that are not divisible neither by 4 nor by 11 were erased. What number is standing at the 1994th place now?
3. On the island of knights and knaves there are three political parties and every native belongs to exactly one of them. Every person was asked the same three questions: are you a member of Party 1? are you a member of Party 2? are you a member of Party 3? Positive answers were given by 60%, 50% and 40% of population respectively. Find out who is in majority in Party 2: knights or knaves?
4. Spam is sold in cans of five types which differ by weight and price (see the pricelist below). There are 1994 cans of spam in the store with total weight 1 ton. Prove that their total price is less than $16000.
| Weight, g | Price, $ |
|---|---|
| 330 | 6 |
| 420 | 7 |
| 550 | 8 |
| 640 | 9 |
| 710 | 10 |
![]()
1. Prove that a third-degree polynomial has to have at least one real root.
2. Prove that if a is a root of f(x) then there exists a polynomial g(x) such that f(x) = (x-a)g(x).
1. A five-digit number A is written with twos and threes only, and a five-digit number B is written with threes and fours only. Is it possible that their product has only twos in its decimal representation?
2. This morning a Petri dish has had 19 blue and 95 red amoebas in it. From time to time they merged and if two red amoebas merged then the result was one blue amoeba; if two blue ones did then the resulting amoeba immediately divided into four red ones; and if a red amoeba and a blue amoeba merged then then result was always three red amoebas. By the end of the day there were 100 amoebas in the dish. How many of them are blue?
3. A special calculator performs the following operation every second: if the number X on its screen is divisible by 2k then the calculator increases X by some natural number from 1 thru k+1 (k here is an arbitrary natural number that divides X). Initially X was set to 1. Prove that each power of 2 will eventually appear on the screen.
4. In triangle ABC point D on AC is such that BD is angle bisector of angle B. Point E is chosen so that angles EAB and ACB are equal and AE = DC. Also it is known that ED and AB intersect at point K. Prove that KE = KD.
![]()
1. Prove that for any non-negative integer k the inequality 2k > k holds true.
2. Prove that for a polynomial f(x) = anxn + ... + a1x1+a0 with integer coefficients and for any its rational root p/q the following is true: an is divisible by q and a0 is divisible by p.
3. Does polynomial f(x) = x4 - 3x3 + x2 - 5x + 7 have any rational roots? Does it have at least one real root?
1. Tom and Jerry play the following game: there is a bar of chocolate with dimensions 17 x 17. One's move consists of breaking one of the bar's rectangular parts into two rectangular parts and after every Tom's move he immediatley eats one of the two newly formed parts. The player who cannot make a move loses. Jerry makes the first move. Who wins in an errorless game?
2. Find all the triples of prime numbers x, y, z such that 19x - yz = 1995.
3. A heirloom consists of several diamonds and is valued at $1,000,000. It is known that it can be divided into five as well as into eight equal parts. What is the maximum possible value of the smallest diamond?
4. Triangle ABC and points D and E on the plane are such that ADB = BEC = 90o. Prove that DE cannot be greater than one half of perimeter of ABC.
![]()
1. In Session 87 HW Question 3: what if we needed to split heirloom into two and three equal parts?
1. Does there exist a polynomial P(x) with integer coefficients such that P(1) = 1492 and P(8) = 2002?
2. Point M is selected on a side BC of isosceles traingle ABC (meaning BC is not the triangle's base) and point N is selected on MC in a such a way that MN = AN. It is known that angles BAM and NAC are equal. Find angle MAC.
3. Represent number 1995 as the sum of maximum possible amount of consecutive natural numbers.
4. Real numbers a, b, and c are such that all three graphs of functions y = ax + b, y = bx + c, y = cx + a have a common point. Prove that a = b = c.
5. Is it possible to denote numbers 1, 2, 3, 4, 5 as a, b, c, d and e in some order so that the equality (a+b)(b+c)(c+d)(d+e)(e+a) = (a+c)(b+d)(c+e)(d+a)(e+b) holds true?
6. Nine rooks are positioned on a 9 x 9 board so that none of them is under attack from another one. After that each one of them was moved using a chess knight's move (two squares in one direction and one square in a perpendicular direction). Prove that now some rook is under attack from some other.
![]()
1. Given any polynomial P(x) with integer coefficients and two integers a and b prove that P(a)-P(b) must be divisible by a-b.
2. Prove that if N rooks stand on a NxN board in such a way that none of them are under attack from any other then an even number of them stand on white squares (presuming standard chess-style coloring with left-lower corner painted black).
1. Polynomials P(x) and Q(x) are such that for any x the two following equalities hold true: P(x) = Q(x2+1) and Q(x) = P(x2-1). Prove that P = Q = const.
2. After a round-robin volleyball tournament in which every team played each other exactly once the teams were split into k groups in such a way that in each group total amount of points gained was the same. (Win is worth 1 point, loss - 0 points; there are no draws in volleyball). Also the first group consists of just one team, second - of two teams, etc., and kth group has k teams. Find all possible values of k.
3. Prove that any convex quadrangle can be dissected into four quadrangles each of them being either a trapezium or a parallelogramm.
4. Do there exist four different natural numbers such that every one of them is divisible by difference of any two other numbers in the quadruple?
![]()
1. Prove that if deg P(x) > 0 then P(x) cannot be constant zero.
2. In triangle ABC angle bisector AF, median BG and altitude CH meet at one point O, and it is known that AB is the longest side of the triangle. Prove that BO > AO.
3. Two thousand letters A and B are written in a row. Prove that the resulting sequence can be split into no more than 800 palindromes (a palindrome is a word - or sequence of letters - that reads the same in both directions; e.g. ABBA).
4. A five-digit number is divisible by 54. One of its digits was crossed out so that the result is again divisible by 54, then another digit was crossed out and the result was again divisible by 54. Finally one other digit was erased and the result was number 54. Find the original number.
![]()
1. Prove that 2001 x 2003+1 is a perfect square.
2. Prove that 1995 x 1997 x 1999 x 2001 + 16 is a perfect square.
3. Prove that any number that differs from 1001 x 1003 x 2003 x 2005 by less than one thousand cannot be a perfect square.
4. Prove that a non-zero polynomial of degree n cannot have more than n roots.
1. Polynomial x3+ax2+17x+3b has three integer roots (a and b are both integers as well). Prove that the roots are all different numbers.
2. Two business partners bought 100 liters of soft drinks in bottles of 0.5 , 0.7 and 1 liter in volume. Then they quarreled and decided to split their possessions evenly. Prove that they can always do that without opening any of the bottles.
3. There are 17 cities in a country of Westland. In every one of them the central square is named in honor of one of the other cities in Westland. A foregner starts his trip in one of the cities A; next day he travels to city B which is the namesake of A's central square, then next day to city C which is the namesake of B's central square, etc. At one moment the traveller realized that he is in the same city he has visited exactly 17 days ago. Prove that he has already visited all the cities in Westland.
4. Points P, Q, R, S are midpoints of sides AB, BC, CD and DA of convex quadrangle ABCD respectively. Point M inside ABCD is such that APMS is a parallelogramm. Prove that CRMQ is a parallelogramm as well.
![]()
1. Prove Viete theorem for quadratic and cubic polynomials.
2. Find derivative for y = x2 at an arbitrary point x = x0.
3. Find the maximum possible area of a rectangle with perimeter 10.
1. Find derivative for y = x3 at x0 = 1.
2. Two natural numbers a and b are such that S(a) = 1993 and S(b) = 1994. Can S(a+b) be equal to 1995? (Here S(x) denotes the sum of all digits of x).
3. Can there be a group of people such that every one of them has exactly five friends among them and every two have exactly two common friends in the group?
4. Two play the following game putting chips on a 101 x 101 board in turn. The first player can put his chip on any empty field such that the overall number of chips already standing in the same row and column with that field is even. The second player can make his move if that number is odd. The player who cannot move loses. Who will win in the errorless game?
![]()
1. Find f'(x) for f(x) = xn.
2. Prove that (kf)' = kf' for any constant k and function f(x).
3. Prove that (f+g)' = f' + g' for any two functions f(x) and g(x).
0. Prove that in Session 92 HW Question 4 regardless of how game progresses the first player always can make a move.
1. Find maximum value of f(x) = x3 - 7x2 + 4x + 1 for 0 <= x <= 7.
2. In a convex quadrangle ABCD angles A and D are equal. Also it is known that perpendicular bisectors for AB and CD intersect at point P which lies on AD. Prove that diagonals AC and BD are equal.
3. At a redemption center one can exchange five empty beer bottles for one bottle of milk, and ten empty milk bottles for one bottle of beer. Jim found 60 empty bottles and used them for redemption and further consumption etc. (the bottles he receives at the redemption center he later uses in similar exchanges). Finally, he is left with one empty beer bottle. How many beer bottles had Jim found initially?
4. Let's call a positive rational number "good" if it cannot be represented as a sum of a few consecutive members of sequence 1/1x2, 1/2x3, 1/3x4, ... Prove that there exist infinitely many "good" numbers that are less than 1/2002.
![]()
1. Use equality 1/n(n+1) + 1/(n+1)(n+2) + ... + 1/m(m-1) = 1/n - 1/m to solve Session 93 HW Question 4.
1. Point M is midpoint of side BC of convex quadrangle ABCD. It is known that angle AMD equals 120o. Prove that AB +(BC/2) + CD >= DA.
2. Several people try to divide the heirloom they inherited. We will call an heir "poor" if he/she gets less than 99 dollars and "rich" if he/she gets more than 10,000 dollars. It is known that no matter how the heirloom is divided all "rich" heirs put together will get no less than all "poor" heirs put together. Prove that in any case all "rich" heirs will get at least 100 times more than all "poor" heirs.
3. One hundred nodes are selected on a sheet of graphed paper. Prove that one can find two of them - say, A and B - such that a rectangle AXBY with sides parallel to the gridlines contains at least 20 selected nodes (counting those lying on the sides and corners).
4. Two different quadratic polynomials f(x) and g(x) both have their leading coefficient equal to 1. Given that f(19) + f(92) = g(19) + g(92) find all the solutions of equation f(x) = g(x).
![]()
1. Prove that in a triangle's angle bisector is always positioned between altitude and median.
2. A cop is chasing a criminal in a park that has the following
shape  . The park's alleys are so dark that
they can see each other only at the distance not greater than a) L/3;
b) L/4; c) L/5, where L is the length of the park's alleys. Prove
that the cop can always catch the criminal if his speed is twice that
of the criminal.
. The park's alleys are so dark that
they can see each other only at the distance not greater than a) L/3;
b) L/4; c) L/5, where L is the length of the park's alleys. Prove
that the cop can always catch the criminal if his speed is twice that
of the criminal.
3. Is it possible to color the squares of infinite sheet of graphed paper into 6 colors so that in any 2 x 3 (or 3 x 2) table all squares have different color?
4. Sum of two non-negative numbers x and y equals 1. What is the minumum and maximum possible value of x3+y3?
![]()
1. Find maximum and minumum values for x3+2y3 where x and y are non-negative numbers with sum equal to 1.
2. Same for 2x3-3y3.
3. What if in Session 95 HW Question 2 cop can see a criminal only if distance between them is less than or equal to L/10. Is it possible that there is no algorithm for the cop which guarantees the capture of the criminal?
1. Two armies - Tips and Tops, one thousand soldiers in each of them - met at the battlefield. First, each of the Tips shot one of the Tops, and then each of the remaining Tops shot a Tip; finally, each of the remaining Tips shot a Top again and the battle ended. Prove that at least 500 soldiers were left alive after the battle (remember that several soldiers can simultaneously shoot at the same enemy)
2. A rectangle is dissected into squares with sides whose lengths
are all whole. Find minimum possible values for the sides of the
rectangle. Here is the dissection diagram: 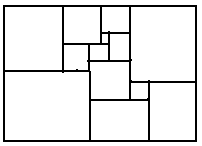
3. Prove that one can find natural numbers x, y, z greater than 20000002 and such that (x2+1)(y2+1) = z2+1.
4. On the island of knights and knaves everybody lives in their own house and some of them are connected with roads so that everybody can drive to at least one other person's place. After a tornado which destroyed some of the roads each of the aborigines said "Now I can reach exactly half of the people I was able to drive to before the tornado". Prove that the knaves constitute at least one third of the island's population.
![]()
1. Prove that "geometric" (ratios in a right triangle) definition of sinx and cosx follows from "circular" (coordinates of a point on a unit circumference) definition of these trigonometric functions.
1. Construct a convex septagon such that each of its diagonals is perpendicular to some other diagonal.
2. Is it possible to find some fifty natural numbers so that their L.C.M. coincides with L.C.M. of some other fifty natural numbers and all these numbers form a set of one hundred consecutive natural numbers?
3. Find minimum positive number x such that [x]{x} >= 3, where [x] and {x} denote integer and fractional parts of x respectively.
4. Naval choirs from one hundred countries are scheduled to participate in a big festival. Each choir is supposed to perform three songs and then immediately depart for home. Upon familiarizing themselves with the songs' lyrics the organizers realized that each song is offensive for some of the participating countries. Prove that they can arrange the order of performances in such a way that nobody will have to listen to more than three songs offensive for their country.
![]()
1. Prove that there is a set of one hundred consecutive natural numbers such that it doesn't contain any prime numbers nor any number divisible by 101 but has two numbers divisible by 97.
1. Nine 10 x 10 squares were dissected into 35 non-square rectangles with integer sides. Prove that it is possible to assemble them into two rectangles with areas that differ by less than 81.
2. AF is median in triangle ABC, D is midpoint of AF, and side AB intersects line CD at point E. Turns out that BD = BF = CF. Prove that AE = DE.
3. The sum of all four roots of two quadratic polynomials f and g with equal leading coefficients is zero. It is known that f+g has two roots. Prove that their sum is also zero.
4. There are 58 members in The Weekend Gourmet Club - every one
of them is either fat or lean. At the last club meeting every fat
club member brought 15 donuts and gave them to the lean members, and
every lean member brought 14 donuts and gave them to the fat members.
It is known that all the fat persons got the same amount of donuts,
and all the lean people received the same amount of donuts as well
(but not necessarily the same amount as the fat ones). How many lean
club members are there? Give all the possible answers.
![]()
1. Prove formulas for sin(x+y), cos(x+y), sin2x, cos2x, etc.
2. Prove that in any triangle r = 2S/p, where r, S, p are inradius, area, and perimeter of the triangle respectively.
3. Prove that in any triangle R = abc/4S, where R, a, b, c, S are circumradius, sides and area of the triangle respectively.
1. Kindergarten children come out of the park in boy-girl pairs carrying acorns they have gathered there. In every pair the number of the boy's acorns is either two times greater or two times fewer than the girl's. Is it possible that they all carry exactly 1000 acorns?
2. Several segments (possibly intersecting) are selected on the straight line. Left half of every one of them is colored red and it turned out that the red points form one contiguous red segment. If instead of coloring left halves red we colored all right halves blue then all blue points would form one contiguous blue segment 10 cm shorter than the red one. Prove that among the original selected segments there are two with lengths that differ by at least 20 cm.
3. Find all pairs of natural numbers m and n such that LCM(m,n) - GCD(m,n) = mn/3
4. Point D is the midpoint of side AC in triangle ABC. Point E on
BC is chosen so that angles BEA and CED are equal. Find ratio AE/DE.
![]()
1. Prove that S = (ab sinC)/2, where S - is area of triangle ABC, a = BC, b = AC.
2. Prove the law of sines.
1. In triangle ABC segments AX and AY are perpendiculars dropped to the bisectors of external angles B and C. Prove that the length of XY equals to half-perimeter of the triangle.
2. Find the minimum natural number such that when divided by any number from 2 thru 10 it produces remainder which is at least half of the number it's being divided by.
3. There are ten sculptures arranged around the circle in the emperor's garden. The emperor ordered to install ten pedestals (one between each two neighboring sculptures) each holding a golden ball with weight equal exactly to the difference between weigths of the two corresponding sculptures. Prove that these ten balls can be divided into two groups so that total weights of the two groups coincide.
4. Two thousand nuts are divided into twenty non-empty piles. It
is allowed to select any two piles A and B such that A has more than
one nut and the number of nuts in A is divisible by the number of
nuts in B, and then carry over one nut from A to B. Prove that using
such operations one can achieve a situation where each pile has at
least three nuts.
![]()
1. Prove that all the altitudes in a triangle meet at one point (orthocenter).
1. In a convex quadrangle ABCD diagonals AC and BD are equal. Also angles BAC and ADB are equal and sum of angles CAD and ADC equals angle ABD. Find angle BAD.
2. There are six infinite arithmetic series (progressions) each consisting of natural numbers. It is known that any one hundred consecutive natural numbers contain at least one member of at least one of the series. Prove that one of the series has common difference no greater than 600.
3. Do there exist natural numbers a, b and c such that (a+b)(b+c)(c+a) = 4242?
4. Octopi living in Mare Imbrium each have one or two friends. This
morning those with two friends became blue and the ones with one friend
became red. It turned out that after that any two friends were colored
differently. Then ten blue and twelve red octopi changed their color, and
after that every two friends have the same color. How many octopi live
in Mare Imbrium?
![]()
1. Find sine and cosine for the following angles: 30o, 45o, 60o, 120o, 300o.
2. Prove that sin(x)/x converges to 1 when x tends to zero.
1. A square yard is fenced all-around and also divided by several other fences into a few smaller square plots of land. All these smaller squares have whole (in yards) sides. Prove that the sum of the lengths of all fences is divisible by 4.
2. In the set N of all natural numbers somehow three mutually disjoint non-empty subsets were selected. Prove that one can find two natural numbers x and y so that they belong to some two different subsets while their sum x+y doesn't belong to the third subset.
3. Three numbers a, b, and c are such that a+b and ab are both divisible by c. Prove that a3-b3 is also divisible by c.
4. In triangle ABC angle B is 60o, AK and CE are angle
bisectors (K and E lie on the corresponding sides of the triangle).
Segments AK and CE meet at point O. Prove that OK = OE.
![]()
1. Prove that in Session 102 HW Question 1 sum of all horizontal fences is equal to the sum of all vertical fences.
1. In a right triangle ABC points M and N on legs AB abd BC respectively are such that AM = CB, BM = CN. Prove that angle between AN and CM is 45o.
2. Find all natural numbers n possessing the following property: if any real numbers x1, x2, ..., xn satisfy equality x1+x2+...+xn = 0 then x1n+x2n+...+ xnn = nx1x2...xn.
3. Prove that there do not exist two trapezoids which are not parallelograms and such that the sides of the first one are equal to the bases of the second and vice versa.
4. After a round-robin volleyball tournament (each team played each other exactly once) it turned out that every team won the same number of matches as all the teams it had defeated put together. How many teams took part in the tournament?
5. Several years after Great South-East empire split into 16 independent duchies it turned out that every duchy is friends with exactly three others and enemies with all the other former "sister-republics". Eight western countries decided to help former GSF members, with each of them helping exactly two duchies which are friends with each other. Is there a guarantee that such a plan is always possible?
6. Does there exist a polynomial with integer coefficients such that its free coefficient is equal to 2001, numbers 1 and 5 are its roots and its value in some integer x is 2000?
7. A public company has 1994 shareholders and it is known that any 1000 of them have controlling interest (that is, they together own at least 50% of the shares). What is the greatest percentage of shares that can be owned by one shareholder?
8. We will call a triangle "non-tall" if at least two of its altitudes are not longer than 1. There are four points on the plane such that any triangle formed by them is non-tall. Prove that there exists a straight line such that distance from any of these points to the line is no greater than 1/2.
9. There are 1000 goblins in the Sauron's army. Every two goblins are either friends or enemies or indifferent to each other. Goblins are incommunicative creatures and talk only to their friends. Also for each goblin every two of his friends are enemies and every two of his enemies are friends. Prove that the army's commander needs to inform at least 200 goblins about the upcoming march for the whole army to learn the news.
10. Dog Encyclopaedia is situated on two shelves in some disarray. The leftmost volume on the upper shelf is "Pooches". Every morning a librarian transposes two volumes with consecutive numbers standing on different shelves. After several days all the volumes have returned back to their original shelves (but perhaps in a different order). Prove that "Pooches" volume is again the leftmost volume on the upper shelf.
11. Prove the following inequality for any real x:
(1+x+x2...+x100)(1+x102) -
102x101 >= 0.
12. For any two consecutive odd numbers p and q prove that pp + qq is divisible by p+q.
13. On a sheet of graphed paper find a figure formed by the minimum possible amount of unit squares and possessing the following quality: if two players play classic game of tit-tat-toe on such a board then the first player has a winning strategy.
14. Numbers u and v are roots of quadratic equation x2+ax+b=0 where a and b are integers. Prove that u100+v100 is also an integer.
15-16. Session 102 Session Question 2 (about sin(x)/x limit) and
Homework Question 2 (about three disjoint sets) - their solutions
need to be finalized as well.
![]()
Back to MathCircle Homepage
Back to DF-ES Homepage