By Crean
Self, Reality, and Mathematics
Goal
It is my will to establish a brief discourse in radical constructivism, and relate this to beliefs of self, specifically with regards to mathematics (and extrapolated to the general teaching arena).
Format
I The Experiment
II Constructivism
III Implications of Constructivism for teaching
IV Self Beliefs (Chapters 6 & 7)
V Should Mathematicians be listening to Cognitive Psychologists
VI Experiment Discussion
Total Estimated Running Time:25 minutes
Note: The constructivist perspective may be considered extremely "revolutionary" in traditional academic arenas. Although constructivism has gained a powerful ally with most modern views of mathematics, it is still rejected by a good portion of traditionalists.
I The Experiment (2 minutes)
II An Introduction to Radical Constructivism (7 minutes)
"Nothing is true; everything is permitted" – Hassan I Sabbah
"…constructivism intends to undermine too large a part of the traditional view of the world. Indeed, one need not venture very far into constructivist thought to realize that it inevitably leads to the contention that man – and man alone – is responsible for his thinking, his knowledge and, therefore, also for what he does. Today, when behaviorists are still intent on pushing all responsibility into the environment, and socio-biologists are trying to place much of it into genes, a doctrine may well seem uncomfortable if it suggests that we have no one but ourselves to thank for the world in which we appear to be living." (Glasersfeld pg. 193)
Knowledge can never be interpreted as a picture or representation of the "real world", but only as a reflection of different pathways to understanding. (Glasersfeld, pg. 194)
The radical comes in when relating to knowledge. "Where as the traditional view of epistemology, as well as of cognitive psychology, that relation is always seen as a more or less picture-like (iconic) correspondence or match, radical constructivism see’s it as an adaptation in the functional sense." (Glasersfeld, pg. 196)
Please think on the full implications of this. In summation, reality is relative, there is no way to know an absolute reality (even if it did exist) so recognize that, only the things to enter into the realm of the experience are to be considered real (if it affects us, it is real). We construct our knowledge as it fits into our world-view.
III Implications of constructivism for teaching (5 minutes)
A model of instruction is proposed with six components (Confrey, pg. 107):
Things to stay away from
"There has recently appeared in increasing amounts of evidence that direct instruction may not provide an adequate base for students’ development and for student use of higher cognitive skills." (Confrey, pg. 107)
Definition: Direct Instruction – Constituent of three parts according to Confrey.
In relation to mathematics: mathematics is not a tool to learn how to use, but a thought process to develop ones cognition (Confrey, 1990).
IV Self Beliefs (Chapters 6) (5 minutes)
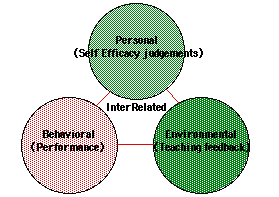
Bandura’s Model of Reciprocal Determinism
A model of a social cognitive learning theory

Personal factors include beliefs and attitudes that affect learning, especially in response to environmental stimuli.
Behavioral factors include responses one makes in a given situation.
Environmental factors include the role of people which are in the environment of the person. (Textbook, ch. 6 pg. 129).
The chapter goes on to elaborate on different aspects of this theory. It’s main emphasis for teaching is to try and exemplify positive attributions and retrain undesirable attributional patterns. The main emphasis of the chapter is on Self Efficacy.
For Definitions and information of Self-efficacy see Modern Cognitive Psychological Texts.
Briefly Re-hashed (to improve efficacy in both students and teachers):
Note: Encourage individual autonomy also. One study has show that "many middle and secondary teachers communicate an authoritarian and thereby limited view of mathematics" (Cooney, 1998). I am sure this is not only in regards to mathematics. One article by Cooney, Shealy, and Arvold dictates that it is highly beneficial to adapt a continual analysis of our personal belief structures as teachers to that we do not get too static in our views. This is in accordance with a constructivist perspective.
V Should Mathematicians be listening to Cognitive Psychologists (3 minutes)
Cognitive psychologists and mathematicians have been found to agree on certain underlying principles of knowledge restructuring (Seldon, 1997). Note that these principles can be abstracted to almost any field of knowledge that is interactive.
Try and observe these when lesson planning, exam planning etc.
VI Experiment Discussion (3 minutes)
Get together with the person next to you and discuss what each of you thought the connections were between the presentation and the beginning experiment.
References (plus the Textbook and Jessica DeCuir's presentation)
Confrey, J. (1990). What constructivism implies for teaching. In R. B. Davis, C. A. Maher & N. Noddings (eds.), Constructivist views on the teaching and learning of mathematics (JRME monograph 4) (pp. 107-122). Reston, VA :NCTM.
Cooney, T; Shealy, E; Briget Arvold. Conceptualizing Belief Structures of Preservice Secondary Mathematics Teachers. Journal for Research in Mathematics Education (1998, Vol 29, No. 3. 306-333).
Glasersfeld, E. An Introduction to Radical Constructivism. (Chapter 10), from the book The Construction of Knowledge (Glasersfeld, E.). Mention of Piaget (1937) La Construction Du Reel Chez l’Enfant. (p. 311).
Green, Thomas F. (1971).The Activities of Teaching. NewYork: McGraw-Hill Book Company. (Chapter 3) Teaching and the Formation of Beliefs.
Selden, John & Annie (1997). Should Mathematicians and Mathematics Educators be listening to Cognitive Psychologists? MAA online (The Mathematical Association of America) Research Sampler. http://www.maa.org/t_and_l/sampler/rs_2.html