Darts is a fabulous sport. You can play it with your friends in local pubs or you can invite the neighbours to a darts-evening. You will never get bored with darts.
Darts is no longer just a pub sport. Millions of darts-fans all over the world have already hung a dart-board on their wall. There is no other play-ground where you can play so many different games as on the Clock-board.
In the following pages you can see some quotations of The Dart Book.
Kari Kaitanen 1996
In this book you can find dozens of different interesting dart-games. Numerous examples with clear pictures welcome all new players to this great sport. You also learn how to throw more accurately.
For experts this book offers a deep and scientific study of darts. For the first time all the finishes of 01-games have been optimised with a computer from every point of the game five turns ahead. Just by knowing the absolute best finish for your level you will greatly improve your chances of winning.
In every sport there are those who win championships. Then there are those who are in it as a hobby. There are many different levels and many ways to throw darts. There are those who make a living with it and those who just enjoy the social life that darts provides - and usually spend their salary in places where they meet. Both players - in my opinion - live a very full life and probably die with a big smile on their face.
For the lesser players, as in every sport, it's almost impossible to beat a master. This is also true in darts. However, it's typical of darts that the game is interesting even if the players are of different levels. With a little luck a beginner can beat an average player. But what's also typical of darts is that there are not many sports where players of different levels can and should do so much so differently from each other. The right choices depend on the level you are playing at. This is if you want to win the game.
It's very useful to watch the decisions masters make and learn from them. But when for example speaking about finishes in 501 (or 301), all the masters have their own favourite finishes. Preferences speak more loudly when you try to find the best 'out', and there is not one objective player in the world to say which 'out' is the best. So this is not the way you can find the best finishes for your level. There is only one way to solve the problem. You must programme the computer to throw with the different - but exact - sprays of darts.
At first we must learn the figure of the dispersion. There is
one old dart-player (there is no historical basis for this), Carl
Friedrich Gauss (1777-1855), who helped us in this enormous task.
Without his discovery of normally diversed phenomenon, it would
be hard to calculate the probabilities exactly. Gauss's dispersion
proved to depict the most natural symptoms in life. So we chose
it to depict the distribution of dart shots.
After solving how the darts are spread around the aiming point you must decide which kind of players you want to take into your calculations. I decided to use four different levels of players. Just to make it easier for you to know what level I am talking about, I have named these levels: professional, expert, average and beginner.
Since the dispersions of darts should be exact for finding the exact percentages for these levels, there must be something that makes them exact. I have chosen the probability of hitting the treble-bed. The 'professional's' probability of hitting the treble is hereby always 46.42% in the book. An expert can hit the treble with the probability of 21.54%. For an average player the same figure is 10.00% and for the beginner only 4.64%.
I have not chosen these numbers randomly: the percentage of a professional raised to the third power makes exactly 10%. You can thus say that a 'pro' can get 180 points - three darts to treble-bed - every tenth time he tries. As you may already have guessed an expert can score 180p every 100th time and so on.
probability of probability of
hitting the scoring 180
treble points
------------------------------------------------------
professional (pro) 46.42% 1 to 10
expert (exp) 21.54% 1 to 100
average (ave) 10.00% 1 to 1000
beginner (beg) 4.64% 1 to 10000
------------------------------------------------------
You must, however, notice that the probability of getting 180
points is only theoretical: (46.4%^3 = 10%). After the first two
darts there is already considerably less space on the treble-bed
for the third dart. Therefore you often have to change your aiming
point. Thus the 'right' probability of scoring the maximum score
may be one-to-twenty or even less.
Then there is the mental pressure, which may be enormous. If you have never reached 180 points before, your hands may start to tremble. If you have practiced darts for many years already and have never scored 180, it is probably the biggest reason for this. It doesn't mean that you must now rank yourself in the 'beginners' group. In fact, even if you try to test the level of your play to find it out, there is no reason to start calling yourself 'an expert' or 'an average player'. As mentioned earlier, the names are used only because it's much easier to understand what kind of sprays I'm talking about. However, the test can help you to see what level you should be interested in.
On the other hand, after the first dart the beginner e.g. might have a good 'sighter' for his next shots, and the probability of throwing the remaining darts into the target can be much bigger than before. So, it's very hard to tell the exact level of scoring 180 points.
It would be more correct to say that an expert can hit
three different trebles, for example T20, T15 and T16, every hundredth
time. Since the theoretical probability is approximately right,
it's easier to say that an expert can get 180 points every tenth
time.
The next task is to iterate the dispersions to receive the exact required percentage. Compared to the first book (published in Finnish) there are many minor improvements. First, the probabilities are calculated much more accurately; instead of using circles of four millimetres, the areas around the aiming point are divided into parts smaller than 0.05 mm. The difference is significant for example in the bull's-eye, the smallest target on the board.
The computer is programmed to imitate the real world as closely as possible. For example, the wires hamper the players. The critical wire width is programmed to be 0.2 mm. This means that an expert aiming at the centre of a treble-bed loses 1.7% of his shots as 'wire-darts'. In the bull's-eye the probability of wire-darts is already 3.1% for the expert.
Note that the wires are, of course, wider than the given 0.2 mm,
but if the dart hits a bit on the side of a wire it still goes
in. It is not possible to know the exact percentage of wire-darts,
but it is important to tell the computer that it's more probable
in some areas - like in the bull's-eye - than in the centre of
singles. Nowadays technology makes darts more flexible and the
percentage of wire-darts is getting smaller.
When the term 'professional player' (pro) is used in the book
it means a player who can throw (theoretically) 180 points every
10th time. The dispersion of darts is thus very small. You can
hear the great 'one-hundred-and-eightyyy...' shouted at the biggest
tournaments often enough to know that this level is not just theoretical.
However, there aren't many players in the world who represent
this honourable level of play.
As you can see in the picture, the area of possible hits is small,
when a 'pro' aims at the centre of a treble-bed. The dispersion
of this level is programmed to the computer, and it uses exactly
the same spray of darts when aiming at any point on the board.
When the dispersion is estimated to be normally divided, you can
calculate the exact probabilities for this level.
The spray of darts is still quite small. If an 'expert player'
(exp) aims at the middle of triple-20, no darts are missed over
sectors 1 and 5. The possibility of throwing 180 points is one
in a hundred (1:100). The player of this level most certainly
plays in a darts-league. Since this level is already represented
by a much greater amount of players - and is still a very high
level - I have tried to mention the 'expert' player often.
The spray of darts around treble-20 already looks more human and
possible to reach than the one of a 'professional player'. Already
16% of his darts fly to the adjacent sectors. The average scoring
is still very high: 74.7 points with three darts (without doubling).
This level can be the result of a great deal of practice and years
of social life in local pubs.
The level of an average player can be very near the next one.
The spray of hits is already quite large. Only one tenth of the
darts hit triple-20 when aiming at the middle of it. Theoretically
an average player (ave) is projected to throw 180 points every
1000th time. A few darts already miss over one sector and when
for example trying the heart of treble-20, one dart in every thirteenth
turn hits sector 18 or sector 12.
Only one tenth of the shots at a treble are successful. Note that
the aiming point often has to be on the other side of a treble,
when the bed is already crowded. Move the same spray of darts
only one centimetre to the right and already every 26th dart (3.8%)
hits sector 18 - meaning one dart every ninth turn. Does this
look more like your play?
Beginners are the group of players where levels vary the most.
But when using the word 'beginner' (beg) in the book, it means
that the spray of darts draws quite a large pancake around the
aiming point. The 'beginner' finds it difficult to hit the same
sector that he aims at and thus the average score is not much
different in any area of the dart-board. 180 points is almost
never achieved (1:10000).
A beginner finds it very frustrating to aim at a double-band.
At first only one shot in fourteen will score. And then there
is the baby in the next room who starts to cry every time you
hit the enclosure cabinet. And these total misses are not few
at the beginning of your road to the championship tournaments.
Just a little practice and you will improve your play a great
deal.
Note that in the 'real world' the dispersion of darts isn't usually exactly a circle, but an ellipse. This due to the way one throws darts at the dart-board. It's often more difficult to release the darts at the same point every time than to keep the same vertical line. This means the spray is more often high than wide. Of course there are players who do the opposite - they are the ones who are more likely to hit the single of sector six (and double-top) than the single-20.
To be more exact the ellipse may not be exactly vertical. For the right-handed players the figure usually leans a bit to the left. This comes from the motion of the hand when throwing. It also gives one more reason to use double-16 (and double-8) in doubling out.
For master players, however, the dispersion is closer to a circle
and for them every area on the dart-board is quite as good.
As you have noticed, everything in darts has something to do with probabilities. When you choose the way of finishing you just try to find the best probability to win the game. The probability of the opponent finishing the next turn is also important. You can estimate these probabilities in your head or you can even calculate them mathematically, as you have seen done in the book first time ever.
At first you must find out the dispersions of darts for the player. You can do that with a test, but that would require a lot of throws to be reliable. Or you can do it purely mathematically when you know the theory of normal dispersion. Since we want to make the results of optimal play as general as possible, we must use a circle as the form of the dispersion. One way or another you have to know what the player's probability of hitting for example a double is, how many darts then fly to a single, which percentage to the sector beside and so on. These probabilities have to be known for every aiming point you want to calculate.
When you have found a suitable spray of darts, you simply move it around the dart-board. When you place exactly the same dispersion to the centre of a double you get the percentage of hitting the double. Note that for the computer there are three aiming points in every bed of treble, double and single.
Naturally, you must also use the same spray of darts for the bull's-eye.
There are many variations for finishing 501. When placing only one aiming point in the middle of each bed: single, double and treble, there are exactly 82407 different ways to end the game! From 58 points you can finish in 1404 different ways! So, if you try to calculate the exact probabilities by hand, you will probably lose a good hand for playing darts.
To be a bit more exact, I chose to use three different aiming points in each bed: one in the middle, and two on both sides of the bed. As there still are 21 points in the bull, the computer uses 201 different aiming points when searching for the optimal play.
Different aiming points are marked a bit differently in the book than you may have seen before. For example 'T16(8)' means the point in treble-16 but closer to sector 8. Marking the aiming points like this also helps you to realise to which sector it is better to miss your dart - if you should miss it.
With the computer we can check every possible way to finish the game. Since the calculating model is quite simple, the programmes are also easy to make. A simple carefully made programme guarantees correct results. All you have to do is to make the computer try every possible aiming point on the dart-board, and search all possible - and sensible - areas the dart might hit. The computer must then try to finish the game from each different point. If it's not programmed to be selective, it has to again try every possible aiming point available. Note that a beginner can very easily hit one side of the board when trying for the other, but this shouldn't affect his choices.
The computer now plays with 201 different aiming points. Then it considers at least 15 different places (treble, double and single - in five sectors) around the aiming points where the dart may land. This makes already 3015 different variations, but for example around the bull's-eye there are 22 beds which the dart may hit. So I now simplify things a bit.
From every possible point it tries to continue the game again - with 201 different ways - and so on. For three darts the computer must thus check at least 27.407.000.000 (3015^3) different variations of play. And after this it has solved an optimised way to finish from only one point. In the game of 501 there are 162 different points where the player can finish the game. This means calculating the probabilities for 4.440.000.000.000 times!
All this requires powerful computers to get results in a reasonable
time. Note that in the book I decided to go a bit further. Like
in chess the computer thinks ahead five turns, i.e. 15 darts!
And the variations above were calculated for three darts only.
There were also more points than 162 to check this time. The computer
searched for the best play from every point under 501! This of
course means that you need to make a bit more complicated programmes
to solve the problems.
There is more. As you have noticed solving the probability of finishing is only one part of the game. But there are always two players in darts and the opponent can finish the game before you. Winning the game means that you must finish first. To find the best way to play the computer must also consider the opponent's moves. When figuring out the optimal finishes, the computer checks the best finishes for every possible situation where the opponent can be. Note that it's assumes that both players play the game with similar skills.
Since the subject is quite interesting, let me show you the way the computer does the calculating. Let's take an example where a professional player has 90 points and three darts in hand. The opponent has 123 points. By playing T20-D15 a pro would still get to the bull with singles (20-20-B50). That is why T20-D15 is easier (47.8%) to finish with three darts than for example T18-D18 (46.5%):
An opponent of the same level is at 123 points and finishes the game in the next turn with the probability of 24.3%. This means that the player can still have a chance to win the game on the second turn with the probability of 75.7% (100%-24.3%). This means that the player will win the game (on the next two turns) with the probability of 78.5% (0.478+0.757·0.405) when trying the path T20-D15. Because the probability for the path T18-D18 is better, 79.7% (0.465+0.757·0.438), in this situation it's better to aim at treble-18.
Simple, isn't it!
Finishing the game of 501 (301) is the most interesting part of the game. As in every sport you can learn a lot by watching how the masters play.
But the problem is that all players have their favourite doubles and finishes from each point. On the other hand, the level of play may be quite different from yours. There is no sense in trying to end the game when it would require a miracle and leads to a worse situation than in normal play.
The difference between several great endings is often small. This gives room for variations of different throws and only the worst ones can be claimed to be wrong. As a conclusion you may say that it's impossible for anyone to give exact rules for how you should finish your game.
It would, however, be interesting to know the exact and best way
to play the game. This is not only for memorising - which of course
gives you a big advantage - but analysing the game is also a very
useful way to improve your own game.
This was the reason to make a computer throw darts. You can programme different dispersions of darts to a computer and thus find the very best finishes for each level of players.
Since the best throw means the best aiming points to win this game - and not finishing on the next turn - you can also see when a player should go for it and when to play it safe. With a more complicated programme you can also include the opponent's situation in the game and find the best throw for every 'real' situation of the game.
There are only a few differences from the real world. For example, due to a lot of practice you may find triple-20 a bit easier target than triple-19. If the difference in probabilities in table is very small, you should choose T20 to play. Better players, like professionals, should be able to hit any part of the board with almost the same success. Since the computer has never 'practiced' darts, it recommends triple-19, even when it is only a little better throw than e.g. triple-20.
At first for a beginner and an average player triple-19 is the
best choice for scoring, but it is assumed that this will pass
with a lot of practice. Therefore the theoretical notation of
the computer - that at bigger points a beginner should use T19
to get points - is removed from the table.
In the following table you will see some results of optimised play in 501. (This table and lots of others are enclosed into The Dart Book. There you can find the optimised play for each point - up to 501pts - also for the second and the last dart in your hand.)
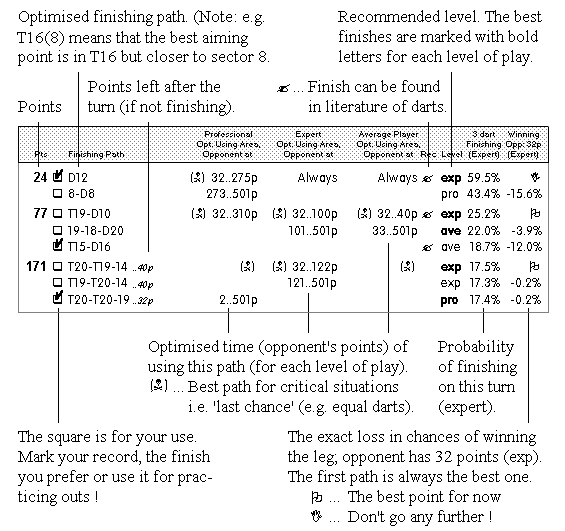
The best paths have been selected by a computer for four different levels of players. Since the area of hits is very large for a beginner, the optimised aiming point might sometimes be in a peculiar place. If for example the sectors 17 and 19 are the right areas to play, the computer may recommend aiming at triple-3. This is no doubt the best place to aim at if the dispersion of darts is big, but since this is in controversy with the basics of darts, I have usually removed these paths from the table. In these cases it's better to practice your throwing - and not start throwing into awkward places on the board. There is a lot of other information hidden in the table.
For every level an exact dispersion is chosen so that exact percentages can be calculated. So please remember that the name of the level only describes the level of players. It doesn't mean that e.g. every 'pro' scores 180 points every tenth time. It only makes the table more understandable.
As mentioned in the book getting to small points doesn't always mean that you have better possibilities to finish the game. The local tops of the winning probabilities (when the opponent has those 32 points) are marked with exlamation marks (in the book it's a small dot).
For maximum accuracy the computer may recommend that you don't use the middle of the bed but one side of it. "T19(7)" means that the best aiming point is in triple-19 but closer to sector 7. With the help of the computer it is possible to find an even more exact point, but it would be more difficult to mark it.
Marking the finishes this way also helps the player to understand the game. You can see that if you miss sector 19, it will be better to avoid sector three and hit sector seven. Moving the aiming point, however, means that you don't hit the best target as often. Since the exact side point is programmed to be 6° (from the centre) to the other side in every bed, the computer may recommend another side for the average player and the centre of the bed for experts. This doesn't mean that the optimal target for an expert is in the centre - but closer to it than to the side point. So, if you read carefully, you also receive information between the lines.
The order of the rows is not random. For each level of player the first path is the best when the exp-level opponent has 32 points (D16). This means the most critical - and perhaps most important - situation. The second path is better than the third one and so on.
Note that there are some points where the outer bull (25p) is the best way to continue the game. But, as proved in the book, the best chance for all levels to hit the outer bull is when you aim at the centre of the bull. (This is when the inner bull doesn't lead you to a bad situation, like busting.) Only if you are better than the 'professional' mentioned in the book, do you start to get an advantage from moving your aiming point to the outer bull. Therefore, if 25 points is the best way to continue the game, the best aiming point is given in parenthesis "B50(25)".
The probabilities of finishing are also listed. There you can find the finishing percentages for the turn (3 darts). The computer optimises your play five turns ahead, so it "plays" the game 15 darts ahead - this means it optimises the game from the very beginning of 501! There are no limits for planning the game even further ahead, but the following turns have little impact on this turn.
Note that the probability of finishing doesn't mean the probability of exactly throwing the mentioned path! That is not important. The probability means the chances of finishing the game when you start the turn by aiming at the given point. All the possible mistakes and the different variations they cause are taken into account in the percentage.
The limits show you at what points the opponent may
be when the path is recommended.
The level of the opponent should now be the same as the player's
- as in most cases it is.
The following table and lots of others are enclosed
into The Dart Book. There you can find the optimised play for
each point (up to 501pts) - also for the second and the last dart
in your hand.
----------------------------------------------------------------------------------
PTS FINISH pro expert average R rec. finish lost
player, player, player, lvl with 3 prob.
use when use when use when darts op32p
oppon. at oppon. at oppon. at (exp) (exp)
----------------------------------------------------------------------------------
170 T20-T20-B50 Always Always Always R pro 0.5% !
169 T20-T20-9...40p (c) (c)32..140p exp -
T20-T20-17...32p 2..501p pro - -0.1%
T19-T20-12...40p 141..501p (c)32..146p ave - -0.1%
T19-T16-T16(8)...16p 147..501p ave - -1.1%
168 T20-T20-8(16)...40p (c)32..109p (c) exp - !
T20-T19-11...40p (c) 110..120p exp - -0.0%
T20-T19-19...32p 32..375p pro - -0.1%
T19-T19-14...40p 121..501p 2..501p ave - -0.2%
167 T20-T19-B50 (c)32..160p (c)32..65p R pro 0.5% !
T19-T20-B50 R pro 0.5% -0.1%
166 T20-T20-14...32p 36..270p 2..501p exp -
T20-T17-15...40p (c)32..82p pro - -0.1%
165 T20-T16-17...40p (c)32..174p (c) exp - !
T19-T20-8(16)...40p 32..140p ave - -0.5%
T19-T19-11...40p 170..501p beg - -0.5%
B50-T19-18...40p (c)32..270p pro - -2.1%
164 T19-T19-B50 32..135p 32..65p pro 0.5% !
T20-T18-B50 (c) (c) R exp 0.5% -0.7%
T19-T19-10...40p 62..348p 2..501p exp 0.0% -1.3%
T20-T16-16...40p (c) ave 0.0% -1.5%
B50-T20-14...40p 131..400p pro - -3.7%
163 T20-T19-14...32p 259..300p 2..501p exp -
T20-T19-6(10)...40p (c) (c) exp - -0.0%
T20-B50-13...40p (c)32..260p pro - -0.4%
T19-T14-T16(8)...16p 32..501p ave - -0.9%
162 T20-T20-10(6)...32p (c)32..93p (c) exp - !
T20-T20-10...32p Always 83..501p exp - -0.0%
T19-T16-17...40p 2..501p ave - -0.7%
161 T20-T17-B50 (c)32..130p (c)32..40p R pro 0.5% !
T20-T19-12...32p 132..135p 33..185p exp 0.0% -0.5%
T20-T19-8(16)...36p (c) ave 0.0% -0.8%
T19-T16-16...40p 2..501p ave 0.0% -1.2%
T19-T19-15...32p 186..501p exp 0.0% -1.2%
B50-T20-19...32p 131..501p pro - -3.7%
160 T20-T20-D20 Always Always Always R exp 1.2% !
T20-B50-B50 R ave 0.3% -5.2%
159 T20-T19-10(6)...32p (c)32..93p (c) exp -
T20-T19-10...32p (c)32..300p 81..501p exp - -0.0%
T19-T14-20...40p 32..501p beg - -0.6%
T20-T17-16...32p 299..501p pro - -1.2%
158 T20-T20-D19 (c)32..410p (c)32..180p (c) R exp 1.2% !
T20-T20-6...32p 181..213p exp 0.0% -4.1%
T19-T19-12...32p 2..501p ave 0.0% -5.3%
157 T20-T19-D20 (c)32..380p Always (c) R exp 1.2% !
T19-T20-D20 2..501p R ave 1.2% -0.7%
156 T20-T20-D18 (c)32..450p (c)32..205p (c) R exp 1.2% !
T19-T19-10(6)...32p 2..501p ave - -4.3%
T19-T19-10...32p 206..338p exp - -4.3%
155 T20-T19-D19 (c)32..350p 32..180p (c) R exp 1.2% !
T19-T20-D19 (c) 32..109p ave 1.2% -0.2%
T20-T19-6...32p 339..440p 181..240p exp 0.0% -4.1%
T19-T14-16...40p 110..501p ave 0.0% -5.3%
154 T19-T19-D20 32..52p 2..501p 2..501p exp 1.2% !
T20-T18-D20 34..341p R pro 1.2% -0.2%
T20-T20-D17 (c) (c) (c) exp 1.2% -0.4%
T18-T20-D20 R pro 1.2% -1.4%
153 T20-T19-D18 (c)32..450p Always Always R exp 1.2% !
152 T20-T20-D16 (c)32..370p Always Always R exp 1.2% !
151 T20-T17-D20 2..501p Always R exp 1.2% !
T19-T18-D20 2..501p R ave 1.2% -0.2%
T20-T19-D17 (c) (c) ave 1.2% -0.4%
T17-T20-D20 R pro 1.2% -2.0%
150 T19-T19-D18 84..450p 2..501p 2..501p exp 1.2% !
T20-T18-D18 32..140p R pro 1.2% -0.3%
T20-T20-D15 (c) (c) (c) R exp 1.2% -0.7%
T20-B50-D20 R exp 0.6% -2.6%
149 T20-T19-D16 (c)32..310p 32..93p (c) R pro 1.2% !
T19-T20-D16 (c)81..501p 2..501p R exp 1.2% -0.1%
148 T20-T16-D20 32..270p 32..220p R exp 1.2% !
T19-T17-D20 (c) exp 1.2% -0.2%
T20-T20-D14 (c) R exp 1.2% -0.6%
T16-T20-D20 219..501p R exp 1.2% -0.8%
T19-T19-D17(2) (c)32..160p ave 1.0% -1.5%
T18-T18-D20 R exp 1.2% -1.9%
147 T19-T18-D18 32..275p 32..160p 32..501p R exp 1.2% !
T20-T17-D18 R pro 1.2% -0.2%
T20-T19-D15 (c) (c) (c) exp 1.2% -0.3%
T19-T16-10...32p 273..310p 199..327p exp 0.0% -3.9%
146 T19-T19-D16 2..501p Always Always R exp 1.2% !
T20-T18-D16 (c) R pro 1.2% -0.7%
145 T20-T15-D20 32..170p 2..501p R exp 1.2% !
T20-T19-D14 (c) R exp 1.2% -0.1%
T19-T16-D20 2..501p ave 1.2% -0.2%
T20-T15(10)-D20 99..246p exp 1.0% -0.6%
T18-T17-D20 448..501p R exp 1.2% -1.9%
T17-T20-D17 (c) (c) exp 1.2% -2.9%
B50-T19-D19 159..450p pro 0.6% -3.1%
144 T20-T20-D12 (c) 32..207p R exp 1.2% !
T20-T16-D18 208..501p R exp 1.2% -0.1%
T19-T17-D18 32..260p pro 1.2% -0.4%
T19-T19-D15 (c) (c)32..48p ave 1.2% -0.4%
T20-T18-D15 R exp 1.2% -0.9%
T19-T19(7)-D15 37..85p ave 1.0% -1.2%
T18-T18-D18 R exp 1.2% -1.9%
B50-T18-D20 261..341p pro 0.6% -3.6%
143 T19-T18-D16 2..501p R exp 1.2% !
T20-T17-D16 (c) R exp 1.2% -0.1%
T20-T19-D13 (c) R exp 1.2% -0.3%
T19-T16-D19 32..64p ave 1.2% -0.3%
T18-T19-D16 32..310p pro 1.2% -1.2%
T17-T20-D16 (c) ave 1.2% -2.1%
T19-T14-12...32p 63..501p ave 0.0% -3.9%
142 T20-T14-D20 32..130p 2..501p R exp 1.2% !
T20-T18-D14 R exp 1.2% -0.4%
T19-T15-D20 R beg 1.2% -0.4%
T20-T20-D11 (c) R exp 1.2% -1.0%
T19-T15(10)-D20 2..501p ave 1.0% -1.0%
T19-T17-D17 (c) exp 1.2% -1.1%
T20-B50-D16 128..129p 90..221p R exp 0.6% -1.5%
T18-T16-D20 R exp 1.2% -1.8%
B50-T20-D16 131..370p pro 0.6% -3.0%
141 T20-T19-D12 (c) 32..183p R exp 1.2% !
T19-T20-D12 32..130p (c) pro 1.2% -0.2%
T20-T15-D18 R exp 1.2% -0.3%
T19-T16-D18 2..501p R ave 1.2% -0.4%
T20-T17-D15 R exp 1.2% -0.6%
T20-T15(10)-D18 184..501p exp 1.0% -0.9%
T17-T18-D18 R exp 1.2% -3.0%
T17-T20-D15 (c) ave 1.2% -3.3%
B50-T17-D20 131..501p pro 0.6% -3.5%
140 T20-D20-D20 (c)32..89p (c)32..60p exp 1.5% !
T20-T20-D10 90..270p 61..130p R exp 1.2% -0.5%
T20-T16-D16 265..501p 145..317p 32..102p R ave 1.2% -0.9%
T20-20-20...40p 128..144p exp 0.2% -3.4%
T19-T17-D16 318..501p (c) ave 1.2% -4.6%
T18-T18-D16 R exp 1.2% -6.1%
T19-19-T16(8)...16p 103..501p ave 0.1% -8.4%
139 T19-T14-D20 32..130p 2..501p 2..501p R exp 1.2%
T20-T19-D11 (c) R exp 1.2% -0.3%
T19-T18-D14 (c) R exp 1.2% -0.4%
T20-T13-D20 R exp 1.2% -1.2%
T19-B50-D16 128..501p 90..221p R exp 0.6% -1.4%
T20-T17-D14 R exp 1.2% -1.5%
T18-T17-D17 (c) exp 1.2% -5.3%
138 T20-D19-D20 32..60p 32..60p exp 1.5% !
T20-T18-D12 61..334p 33..120p R exp 1.2% -0.4%
T20-D20-D19 (c) (c) exp 1.5% -0.6%
T20-T20-D9 R exp 1.2% -0.8%
T20-T14-D18 335..450p R exp 1.2% -1.3%
T19-T19-D12 2..501p R ave 1.2% -1.6%
T18-T20-D12 131..170p pro 1.2% -1.7%
T19-T17-D15 (c) ave 1.2% -2.1%
T20-20-18...40p 121..501p exp 0.2% -3.3%
T18-B50-D17 159..221p pro 0.6% -3.4%
137 T20-T19-D10 33..270p 32..143p R exp 1.2% !
T19-D20-D20 (c)32..40p (c) exp 1.5% -0.5%
T20-T15-D16 262..315p R exp 1.2% -1.1%
T19-T16-D16 2..501p R ave 1.2% -1.4%
T17-T18-D16 311..501p R pro 1.2% -2.9%
T20-19-18...40p 144..501p exp 0.1% -3.2%
T18-T17-D16 (c) ave 1.2% -3.3%
136 T20-D18-D20 32..60p 32..40p exp 1.5% !
T20-D20-D18 (c) (c) exp 1.5% -0.2%
T20-T20-D8 61..334p 33..130p R exp 1.2% -0.3%
T20-T16-D14 R exp 1.2% -0.4%
T19-T19-D11 82..97p 32..48p ave 1.2% -0.6%
T19-T17-D14 (c) ave 1.2% -1.8%
T20-20-16...40p 141..501p 55..88p ave 0.2% -3.2%
T19-20-19...40p 91..140p 33..501p ave 0.0% -3.6%
135 T19-D19-D20 32..60p R exp 1.5% !
T19-T18-D12 265..334p 33..52p R exp 1.2% -0.4%
T20-T17-D12 95..270p 61..160p R exp 1.2% -0.5%
T20-T15-D15 R exp 1.2% -1.0%
T19-T14-D18 335..450p 36..501p ave 1.2% -1.3%
T20-T13-D18 R exp 1.2% -2.0%
T18-T17-D15 (c) ave 1.2% -3.0%
T19-19-19...40p 159..501p 32..139p ave 0.1% -3.4%
B50-T15-D20 32..100p R pro 1.6% -5.5%
B50-T19-D14 (c) (c) R pro 1.6% -5.6%
134 T20-D17-D20 32..60p 32..40p exp 1.5% !
T20-T14-D16 61..310p 33..501p R exp 1.2% -0.0%
T20-D19-D18 (c) pro 1.5% -0.2%
T19-T19-D10 32..74p R ave 1.2% -0.3%
T20-T18-D10 R exp 1.2% -0.5%
T18-D20-D20 (c) exp 1.5% -0.9%
T19-T19-D10(6) 75..92p ave 1.0% -1.1%
T19-T15-D16 R ave 1.2% -1.4%
T17-T17-D16 302..410p (c) pro 1.2% -2.8%
T19-19-18...40p 91..501p beg 0.1% -3.4%
133 T19-D18-D20 32..60p 32..40p exp 1.5% !
T20-T19-D8 61..354p 33..198p R exp 1.2% -0.1%
T19-D20-D18 (c) beg 1.5% -0.2%
T19-T20-D8 R pro 1.2% -0.3%
T20-T11-D20 355..501p R exp 1.2% -1.1%
T14(11)-T17-D20 (c) exp 1.6% -2.8%
T14(11)-T19-D17 (c) ave 1.6% -3.1%
T19-20-16...40p 2..501p ave 0.2% -3.1%
T20-17-16...40p 199..501p exp 0.1% -3.4%
132 T20-D20-D16 261..501p 2..501p exp 1.5% !
T20-T16-D12 211..260p 33..260p R exp 1.2% -0.1%
T20-T12-D18 R exp 1.2% -0.5%
T19-T17-D12 95..210p 82..99p exp 1.2% -0.6%
T20-T20-D6 R exp 1.2% -0.8%
T19-T19-D9 32..40p ave 1.2% -1.0%
T18-T18-D12 R exp 1.2% -1.5%
T17-T17-D15 (c) ave 1.2% -3.7%
T19-19-16...40p 33..501p ave 0.1% -3.7%
B50-T14-D20 32..100p R pro 1.6% -4.2%
B50-T18-D14 (c) R pro 1.6% -4.4%
B50-T20-D11 (c) exp 1.6% -4.7%
B50-B50-D16 R pro 1.3% -4.9%
131 T19-D17-D20 32..60p 32..40p exp 1.6% !
T19-T14-D16 33..501p R exp 1.3% -0.0%
T19-D19-D18 (c) pro 1.6% -0.2%
T20-T13-D16 61..501p R exp 1.2% -0.2%
T20-T17-D10 R exp 1.2% -0.8%
T14(11)-T19-D16 (c) (c) exp 1.6% -0.9%
T20-T19-D7 R exp 1.3% -1.2%
T19-18-16...40p 2..501p ave
130 T20-T20-D5 (c)32..130p (c)32..40p R pro 3.8% !
T20-20-B50 R exp 3.4% -0.9%
T20-T14-D14 35..48p exp 2.5% -1.3%
T20-T10-D20 R exp 2.5% -1.3%
T20-T18-D8 125..129p R exp 2.5% -1.6%
T19-T19-D8 131..354p 33..197p 32..77p R exp 1.2% -3.1%
T14(11)-T20-D14 (c) ave 1.6% -4.1%
T19-17-16...40p 198..501p exp 0.1% -6.3%
T19-14-19...40p 78..501p ave 0.0% -6.5%
129 T19-D20-D16 (c)32..65p (c)32..40p pro 2.8%
T19-T16-D12 62..89p 33..60p 32..501p R pro 2.5% -0.1%
T20-T19-D6 79..135p R pro 2.5% -0.4%
T19-T12-D18 R pro 2.5% -0.5%
T19-T20-D6 R pro 2.5% -0.8%
T20-19-B50 R exp 2.1% -2.0%
T20-T15-D12 61..67p exp 1.2% -2.1%
T20-T11-D18 R exp 1.2% -2.1%
T20-T15(10)-D12 68..80p exp 1.0% -2.6%
T20-T13-D15 R exp 1.2% -2.7%
T18-T17-D12 131..310p 81..160p exp 1.2% -3.1%
T14(11)-T19-D15 (c) ave 1.6% -3.4%
128 T20-D18-D16 32..56p exp 2.3%
T18-D17-D20 32..60p pro 2.8% -0.6%
T18-T14-D16 61..130p R pro 2.5% -0.6%
T18-D19-D18 (c) pro 2.8% -0.8%
T18-T18-D10 R pro 2.5% -1.1%
T18-D20-D17 (c) exp 2.8% -1.2%
T20-18-B50 R exp 2.2% -1.2%
T19-T13-D16 125..501p 61..501p 85..501p R ave 1.3% -1.3%
T20-T12-D16 33..60p exp 1.2% -1.3%
T20-T16-D10 R exp 1.2% -1.8%
T20-T12(9)-D16 32..84p ave 1.0% -1.8%
T20-T20-D4 R exp 1.2% -1.8%
T15(10)-T17-D16 (c) ave 1.6% -2.8%
127 T20-T17-D8 (c)32..160p (c)32..60p R pro 3.8% !
T20-T9-D20 61..67p exp 2.5% -1.7%
T20-17-B50 R exp 3.3% -1.9%
T19-20-B50 R exp 2.2% -3.3%
T19-T14-D14 32..501p ave 1.2% -3.7%
T19-T10-D20 R exp 1.2% -3.7%
T19-T18-D8 R exp 1.2% -4.0%
T16-T19-D11 63..97p exp 1.2% -4.7%
T15(10)-T14-D20 221..501p exp 1.6% -4.7%
T18-T19-D8 159..354p pro 1.2% -5.3%
T15(10)-B50-D16 201..220p exp 1.1% -5.7%
T9-T20-D20 (c) ave 1.6% -7.2%
T16-20-19...40p 91..200p exp 0.0% -7.4%
126 T19-T19-D6 (c)32..135p (c)32..60p R pro 3.8% !
T19-19-B50 R exp 3.3% -1.6%
T19-T15-D12 61..67p R exp 2.5% -1.7%
T19-T11-D18 2..501p R ave 2.5% -1.7%
T19-T15(10)-D12 68..92p exp 2.3% -2.1%
T19-T11(14)-D18 33..134p ave 2.3% -2.2%
T16-T18-D12 R exp 2.5% -3.1%
T16-T14-D18 R beg 2.5% -3.9%
T20-16-B50 R exp 2.2% -4.4%
T20-D15-D18 R exp 1.5% -5.0%
T18-D20-D16 261..501p pro 1.5% -5.1%
T14-T20-D12 91..205p exp 1.4% -5.1%
T18-T16-D12 256..260p pro 1.2% -5.1%
T14-T16-D18 206..501p exp 1.4% -5.2%
T20-T12-D15 R exp 1.2% -5.3%
T20-T18-D6 R exp 1.2% -5.4%
T15(10)-T17-D15 (c) ave 1.6% -5.5%
T17-T17-D12 131..255p pro 1.2% -5.8%
125 B50-T17-D12 (c)32..310p (c)32..61p (c) R pro 3.0% !
T20-B50(25)-D20 62..89p R exp 2.4% -1.0%
B50-B50(25)-B50 R exp 2.9% -1.2%
T20-T15-D10 R exp 2.6% -1.8%
T18-T13-D16 R exp 2.5% -2.9%
T20-T11-D16 88..200p R exp 1.3% -3.4%
T20-15-B50 R exp 2.1% -3.6%
T20-T19-D4 R exp 1.3% -3.8%
T15-T16-D16 R exp 2.7% -4.0%
T19-T12(9)-D16 204..501p 2..501p ave 1.1% -4.6%
124 T20-T14-D11 (c)32..130p (c)32..40p R pro 3.8%
T20-T8(16)-D20 32..82p ave 2.8% -0.6%
T19-T17-D8 125..160p 33..60p R exp 2.5% -0.9%
T20-T16-D8 R ave 2.7% -1.0%
T20-14-B50 R ave 3.4% -1.4%
T20-T12-D14 R ave 2.5% -1.9%
T20-T20-D2 R exp 2.5% -2.4%
T19-T9-D20 159..227p 61..100p exp 1.2% -2.6%
T16-T20-D8 228..350p 91..136p exp 1.4% -2.8%
T14(11)-T18-D14 (c) ave 2.6% -3.0%
T18-T18-D8 R exp 1.2% -4.7%
T16-20-16...40p 134..501p 180..501p ave 0.3% -5.5%
T20-16(8)-8(16)...40p 83..97p ave
T19-11-16...40p 98..179p ave
123 T19-T16-D9 (c)32..100p (c)32..40p R pro 3.8% !
B50-T19-D8 95..354p 33..60p exp 3.1% -0.2%
B50-T17-D11 (c) ave 3.1% -0.5%
T20-T13-D12 61..77p R exp 2.5% -1.0%
T19-16-B50 R beg 3.4% -1.3%
T19-T14-D12 32..133p R ave 2.5% -1.7%
T19-T10-D18 R ave 2.5% -1.8%
T20-T9-D18 75..263p exp 1.2% -3.4%
T17-D20-D16 378..400p 264..501p exp 1.5% -5.1%
T19-10-16...40p 134..501p ave
122 B50-D20-D16 261..380p 32..40p (c) exp 3.2% !
B50-T16-D12 90..260p 33..60p R exp 3.1% -0.0%
T19-B50(25)-D20 61..89p 32..40p exp 2.4% -1.0%
T18-D18-D16 R exp 3.6% -2.5%
T18-T18-D7 (c)32..89p (c) R pro 3.8% -2.8%
T19-T11-D16 88..240p 33..211p ave 1.3% -3.3%
T18-18-B50 R exp 3.5% -3.7%
T18-T12-D16 R exp 2.6% -3.7%
T20-D15-D16 R exp 1.6% -3.8%
T20-T10-D16 381..501p R exp 1.3% -3.9%
T18-T20-D4 R exp 2.6% -4.2%
T16-T14-D16 239..501p exp 1.5% -4.7%
T14-T16-D16 212..501p ave 1.6% -5.0%
T12-T18-D16 R exp 2.8% -5.9%
121 T20-B50(25)-D18 32..40p R exp 3.6% !
B50-T13-D16 95..501p 33..103p exp 3.1% -0.4%
T20-T11-D14 (c)32..100p R pro 3.8% -0.6%
B50-T17-D10 (c) ave 3.1% -0.7%
T20-T7-D20 R exp 2.7% -2.1%
T19-T8(16)-D20 201..237p 32..501p ave 1.7% -2.1%
T20-11-B50 R exp 3.3% -2.4%
T20-T15-D8 R exp 2.5% -2.4%
T19-T16-D8 R exp 1.6% -2.5%
T19-D16-D16 238..501p exp 1.7% -2.8%
T17-T20-D5 (c) R pro 3.8% -2.9%
T17-20-B50 R exp 3.4% -3.8%
T16-T19-D8 102..200p exp 1.6% -3.9%
T17-T10-D20 R exp 2.5% -4.1%
T17-T18-D8 R exp 2.5% -4.4%
120 T20-20-D20 Always (c)32..248p Always R exp 8.1% !
T20-D14-D16 249..501p exp 4.7% -8.0%
119 T19-T12-D13 (c)32..130p (c)32..40p R pro 5.9%
T20-19-D20 125..501p 33..501p R exp 5.0% -0.2%
T19-12-B50 R beg 5.5% -1.3%
T19-T10-D16 R ave 4.6% -1.5%
T19-T10(6)-D16 32..154p ave 4.4% -2.0%
19-T20-D20 (c) ave 5.1% -2.6%
118 T20-18-D20 Always Always (c)32..80p R exp 8.2% !
T18-T16-D8 R ave 4.5% -8.5%
T18-14-B50 R ave 5.3% -8.8%
T19-19-10(6)...32p 79..501p ave
117 T20-17-D20 33..501p 2..501p 2..501p R exp 8.1% !
T19-20-D20 (c)32..40p (c) (c) R beg 8.5% -0.4%
116 T19-19-D20 (c)32..315p Always 2..501p R exp 8.3% !
T20-16-D20 311..501p (c) R exp 8.3% -0.7%
T20-20-D18 R exp 8.2% -1.8%
115 T19-18-D20 33..501p 2..501p Always R exp 8.4%
T20-15-D20 32..40p R exp 8.4% -0.4%
T20-17-D19 (c) (c) exp 8.4% -1.9%
T15-20-B50 R exp 5.5% -9.4%
114 T19-17-D20 32..40p 32..40p 2..501p ave 8.4%
T20-14-D20 33..501p 33..501p R exp 8.2% -0.0%
T20-18-D18 R exp 8.2% -0.9%
T20-16-D19 (c) ave 8.2% -1.5%
T19-19-D19 (c) (c) exp 8.5% -1.7%
113 T19-16-D20 32..310p (c)32..60p (c)32..154p R pro 8.6% !
T20-13-D20 302..501p 61..501p R exp 8.3% -0.4%
T20-17-D18 R exp 8.5% -0.9%
T19-18-D19 (c) pro 8.6% -1.6%
T19-16(8)-D20 155..501p beg 7.6% -2.4%
112 T20-12-D20 2..501p 32..40p R exp 8.5% !
T20-20-D16 2..501p 170..254p R pro 8.5% -0.2%
T20-16-D18 (c) (c) (c) ave 8.6% -0.5%
T19-15-D20 33..501p ave 8.3% -0.9%
111 T19-14-D20 2..501p 2..501p R exp 8.5% !
T20-11-D20 R exp 8.4% -0.6%
T20-19-D16 2..501p R pro 8.4% -0.8%
T19-18-D18 (c) (c) R exp 8.6% -0.9%
T19-16-D19 (c) ave 8.6% -1.4%
T17-20-D20 R pro 8.1% -2.2%
110 T19-13-D20 2..501p 2..501p 32..138p exp 8.6%
T18-16-D20 90..270p R pro 8.5% -0.2%
T20-10-D20 R exp 8.5% -0.4%
T19-17-D18 (c) (c) exp 8.7% -0.5%
T20-18-D16 271..415p R pro 8.4% -0.7%
T20-16-D17 (c) ave 8.5% -2.1%
T14-T12(9)-D16 139..501p ave 4.7% -9.1%
T20-B50 R ave (2 darts)
109 T20-9-D20 2..501p R exp 8.4% !
T19-12-D20 2..501p R ave 8.5% -0.2%
T20-17-D16 2..501p R pro 8.4% -0.2%
T19-20-D16 R exp 8.5% -0.4%
T17-18-D20 (c) exp 8.5% -0.5%
T19-16-D18 (c) (c) ave 8.5% -0.7%
T14-T9-D20 75..99p ave 5.7% -7.3%
108 T20-8(16)-D20 32..140p Always exp 9.1% !
T20-16(8)-D16 141..152p exp 9.1% -0.1%
T16-20-D20 (c)32..90p (c) pro 9.1% -0.4%
T20-8-D20 R exp 8.9% -0.6%
T20-16-D16 R pro 8.8% -0.7%
T19-11-D20 153..501p exp 8.4% -1.4%
T19-19-D16 91..501p 161..240p R pro 8.4% -1.6%
T18-18-D18 R pro 8.8% -3.4%
107 T19-10-D20 32..283p 85..160p R exp 9.3% !
T19-18-D16 32..347p R pro 9.2% -0.2%
T19-14-D18 (c) pro 9.2% -0.6%
T19(7)-10-D20 32..84p ave 8.8% -1.3%
T19-16-D17 (c) exp 9.3% -1.6%
T19-10(6)-D20 161..501p ave 8.2% -2.3%
T20-7-D20 R pro 8.7% -2.6%
T19(7)-16-D17 (c) ave 8.8% -2.6%
T18-13-D20 284..501p exp 8.4% -2.6%
T20-15-D16 339..501p R pro 8.4% -3.0%
T17-16-D20 R pro 8.7% -3.2%
T19-B50 R beg (2 darts)
106 T20-6(10)-D20 32..77p (c) exp 9.1%
T16-18-D20 (c) 63..99p 32..40p exp 9.0% -0.2%
T20-10(6)-D18 (c) exp 9.1% -0.2%
T20-6-D20 R exp 8.9% -0.5%
T20-14-D16 61..501p 81..501p R pro 8.5% -0.9%
T20-10-D18 R exp 8.9% -1.0%
T17-15-D20 32..60p pro 8.6% -1.1%
T18-12-D20 119..141p exp 8.4% -1.3%
T14-T16(8)-D8 48..72p ave 6.0% -6.2%
T14-T8(16)-D20 33..84p ave 6.0% -6.3%
105 T19-8(16)-D20 (c)2..501p exp 9.6% !
T19-16(8)-D16 130..348p exp 9.5% -0.1%
T16-17-D20 32..60p 61..137p 2..501p ave 9.4% -0.3%
T19-8-D20 R exp 9.3% -0.6%
T19-16-D16 61..501p R pro 9.2% -0.7%
T19(7)-8(16)-D20 (c) ave 9.1% -1.6%
T20-13-D16 R pro 8.9% -1.7%
T16-19-D19 (c) pro 9.4% -1.9%
T20-5-D20 R pro 8.9% -2.0%
T15-20-D20 R pro 8.7% -3.1%
104 T16-16-D20 32..40p 32..220p (c)32..156p exp 9.5% !
T19-7(19)-D20 (c) exp 9.7% -0.6%
T19-15-D16 33..100p pro 9.1% -1.3%
T16-18-D19 (c) pro 9.5% -1.5%
T20-4-D20 R exp 8.8% -1.9%
T18-10-D20 221..501p R pro 9.0% -2.1%
T20-12-D16 R pro 8.5% -2.2%
T16-16(8)-D20 157..501p ave 8.5% -2.3%
T18-18-D16 99..501p R pro 8.9% -2.3%
T18-B50 R ave (2 darts)
103 T19-6(10)-D20 32..80p Always exp 10.1% !
T19-10(6)-D18 (c) exp 10.1% -0.2%
T19-6-D20 40..40p R exp 9.8% -0.5%
T19-14-D16 32..89p 81..224p R pro 9.5% -0.9%
T19-10-D18 (c) R exp 9.8% -1.0%
T20-3-D20 R pro 9.5% -1.6%
T20-11-D16 225..501p R pro 8.9% -2.7%
T17-12-D20 R pro 9.2% -2.9%
T17-20-D16 90..501p R pro 9.1% -3.1%
102 T20-10(6)-D16 (c)32..100p exp 9.6%
T14-20-D20 (c)32..65p 2..501p R ave 9.6% -0.3%
T20-10-D16 95..104p R exp 9.3% -0.5%
T19-13-D16 62..501p 105..501p R pro 8.9% -1.2%
T20(5)-10(6)-D16 (c) ave 9.2% -1.4%
T20-2-D20 R pro 9.0% -1.4%
101 T19-4(18)-D20 32..40p exp 10.1% !
T19-4-D20 34..48p 32..32p exp 9.9% -0.1%
T19-12-D16 33..190p 33..80p R exp 9.5% -0.3%
T19-16(8)-D14 R beg 10.1% -0.4%
T19-8-D18 R ave 9.9% -0.5%
T19-6(10)-D19 (c) ave 10.1% -0.9%
T19-10(6)-D17 (c) exp 10.1% -0.9%
T20-9-D16 R pro 9.4% -1.0%
T18-7-D20 40..40p pro 9.7% -1.1%
T19-16-D14 R exp 9.8% -1.2%
T20-1-D20 R pro 9.4% -1.3%
T16-13-D20 81..501p ave 9.4% -1.3%
T18-15-D16 2..501p pro 9.4% -1.5%
T14-19-D20 (c) beg 9.6% -2.0%
T17-10-D20 R pro 9.1% -2.3%
T17-18-D16 R pro 9.0% -2.5%
T15(10)-16-D20 191..501p exp 8.7% -3.1%
T17-B50 R ave (2 darts)
100 T20-D20 Always Always Always R exp 14.6% !
99 T19-10(6)-D16 (c)32..100p Always exp 10.8%
T19-10-D16 (c)32..270p 95..230p R pro 10.5% -0.4%
T19-2-D20 R pro 10.2% -1.4%
T16-11-D20 264..501p exp 9.4% -3.9%
T16-19-D16 268..501p 231..263p pro 9.3% -4.0%
98 T20-D19 (c)32..255p (c)32..120p (c)32..83p R exp 13.2%
T20-6-D16 254..315p 119..197p exp 10.7% -4.2%
T18-12-D16 R ave 10.3% -5.3%
T14-16-D20 84..157p ave 9.7% -7.0%
T16-10-D20 198..501p exp 9.6% -7.1%
T16-18-D16 311..501p 199..207p exp 9.5% -7.3%
T14-16(8)-D20 158..501p ave 8.7% -9.1%
T16-B50 R beg
97 T19-D20 Always Always Always R exp 15.1% !
96 T20-D18 (c)32..376p (c)32..286p (c)32..104p R exp 15.1%
T16-8(16)-D20 105..501p ave 10.9% -7.2%
T20-4-D16 377..501p 287..501p exp 10.7% -8.0%
T19-7-D16 131..180p exp 9.8% -8.3%
95 T19-D19 (c)32..170p (c)32..100p (c)32..120p R exp 13.2%
T19-6(10)-D16 119..127p ave 10.9% -3.8%
T20-3-D16 159..315p 99..501p R exp 10.1% -4.8%
B50-13-D16 R beg 10.1% -8.5%
T15-B50 R ave
94 T18-D20 (c)32..450p (c)2..501p (c)2..501p R exp 15.1%
T20-D17 101..105p exp 12.6% -3.7%
T14-12-D20 156..183p ave 10.6% -8.1%
T20-2-D16 106..175p exp 9.6% -8.2%
B50-12-D16 R beg 10.1% -11.0%
93 T19-D18 (c)32..376p (c)32..287p Always R exp 14.4% !
T19-4-D16 377..501p 288..501p exp 10.0% -7.9%
T20-1-D16 159..260p pro 9.5% -9.1%
B50-3-D20 R beg 9.9% -11.0%
B50-11-D16 R beg 9.6% -11.5%
92 T20-D16 Always Always Always R exp 15.1% !
B50-10-D16 R beg 10.3% -11.0%
91 T17-D20 (c)32..272p Always (c) R exp 14.3%
T19-D17 32..59p ave 13.5% -3.5%
T19-D17(2) 37..80p ave 13.0% -4.3%
T14(11)-13-D18 81..173p ave 10.8% -7.0%
T18-5-D16 271..350p pro 9.5% -9.6%
B50-9-D16 R beg 10.1% -10.7%
90 T18-D18 79..375p 32..103p (c)32..48p R exp 15.2%
T20-D15 (c)32..88p (c) R pro 15.8% -1.9%
B50-D20 R beg 12.4% -4.5%
T14(11)-8(16)-D20 44..56p ave 11.6% -5.6%
T14-8(16)-D20 33..501p ave 11.6% -5.9%
T16-10-D16 102..301p exp 10.2% -7.3%
T17-7-D16 159..260p pro 9.8% -8.8%
89 T19-D16 (c)32..170p (c)32..89p Always R exp 17.8% !
T15(10)-12-D16 84..134p exp 10.5% -9.3%
T18-3-D16 130..501p exp 10.1% -10.7%
T16-9-D16 159..310p pro 9.8% -11.1%
88 T20-D14 (c)32..130p (c)32..40p R pro 16.9%
T16-D20 125..450p 33..501p Always R exp 15.5% -0.3%
T14(11)-14-D16 121..177p exp 10.5% -7.6%
87 T17-D18 (c)32..170p (c)32..65p (c)32..40p R exp 17.6%
T15(10)-10(6)-D16 33..501p ave 11.6% -7.0%
T15(10)-10-D16 259..501p exp 11.4% -7.3%
T14-13-D16 62..258p exp 10.8% -7.7%
T20-11-D8 159..220p pro 12.6% -8.3%
D20-15-D16 221..501p pro 12.2% -8.9%
86 T18-D16 (c)32..250p (c)2..501p (c)2..501p R exp 17.0% !
T14-12-D16 55..135p ave 11.4% -8.1%
D19-16-D16 251..501p pro 12.7% -8.7%
T12(9)-10-D20 99..160p exp 10.6% -9.0%
T12(9)-18-D16 161..207p exp 10.5% -9.2%
85 T15-D20 (c)32..130p (c)32..40p (c) R pro 18.1% !
T19-D14 R pro 17.9% -0.4%
T15(10)-D20 2..501p ave 17.0% -0.9%
B50-3-D16 128..501p 33..130p exp 15.8% -1.0%
B25(Left)-20-D20 R exp 15.0% -3.7%
T14(11)-11-D16 128..501p exp 10.6% -9.1%
84 B50-D17 99..220p 32..108p exp 17.1% !
T16-D18 (c)32..40p (c) 2..501p R ave 17.8% -0.5%
T20-D12 33..100p (c) R pro 17.7% -1.3%
B50-2-D16 221..501p 109..205p exp 15.7% -2.2%
T18-D15 R exp 16.0% -5.2%
T12(9)-16(8)-D16 206..501p exp 11.6% -9.6%
83 T17-D16 (c)32..372p (c)32..48p (c) R pro 17.7%
B50(25)-18-D20 33..143p exp 16.2% -1.1%
T19-D13 32..60p ave 16.2% -3.8%
T9(12)-16-D20 144..257p exp 11.0% -9.2%
T12(9)-15-D16 258..501p exp 10.5% -9.7%
B50-1-D16 131..323p pro
82 B50-D16 2..501p (c)32..260p R exp 18.3% !
T14-D20 (c) Always R ave 18.0% -1.9%
B25(Down)-17-D20 R exp 17.3% -3.0%
T18-D14 R pro 17.8% -4.3%
T11(14)-9-D20 281..501p exp 13.8% -7.8%
T11(14)-17-D16 259..280p exp 13.8% -7.9%
81 T19-D12 (c)32..130p (c)32..47p 32..103p R pro 18.2%
T15-D18 (c) R ave 18.1% -0.4%
B50-7(19)-D12 33..60p exp 16.4% -1.2%
B50-7-D12 61..195p exp 16.3% -1.3%
T15(10)-D18 115..501p ave 17.1% -1.4%
T17-D15 R ave 16.0% -5.1%
T10(15)-11-D20 286..501p exp 14.0% -5.6%
T10(15)-19-D16 268..501p 196..285p exp 14.0% -5.7%
T14(11)-7-D16 102..121p ave 15.1% -5.9%
B50(25)-16-D20 131..270p pro
80 T20-D10 (c)32..310p (c)32..89p R exp 24.0% !
20-20-D20 90..260p (c)32..64p ave 21.7% -2.7%
T16-D16 63..501p R ave 18.8% -9.9%
20-D14-D16 261..501p exp 8.2% -23.9%
79 T19-D11 32..300p (c)32..60p (c) R pro 23.0%
19-20-D20 32..189p ave 21.4% -1.4%
20-19-D20 299..501p 61..501p exp 21.1% -1.6%
T13-D20 (c) R pro 17.9% -10.2%
T17-D14 R pro 17.8% -11.2%
T11-6(10)-D20 190..501p ave
78 T18-D12 (c)32..315p (c)32..103p R exp 24.0% !
20-18-D20 102..501p (c)32..130p ave 21.8% -2.9%
T14-D18 131..501p R ave 19.6% -10.3%
T16-D15 R pro
77 T19-D10 (c)32..310p (c)32..100p (c)32..40p R exp 25.2% !
19-18-D20 101..501p 33..501p ave 22.0% -3.9%
T15-D16 R ave 18.7% -12.0%
76 T16-D14 (c)32..60p (c)32..60p pro 25.8% !
T20-D8 61..400p 61..121p R exp 24.1% -1.3%
20-16-D20 122..501p (c)32..164p ave 22.0% -4.1%
20-16(8)-D20 165..501p beg 17.9% -10.4%
75 T17-D12 (c)32..270p (c)32..116p R exp 24.4%
T19-D9 (c) ave 23.4% -2.2%
T15-D15 R exp 23.2% -2.3%
19-16-D20 117..501p 32..164p ave 21.9% -3.3%
19-16(8)-D20 165..501p beg 17.8% -9.7%
B50(25)-18-D16 271..501p pro 18.6% -9.7%
T13-D18 R pro
74 T14-D16 Always (c)32..260p (c)32..59p R exp 26.0% !
18-16-D20 261..501p 33..164p ave 22.2% -5.7%
18-16(8)-D20 165..501p ave 18.1% -11.9%
73 T19-D8 (c)32..360p (c)32..120p (c)32..40p R exp 25.6%
19-14-D20 33..140p ave 22.3% -4.3%
17-16-D20 119..501p 141..164p ave 21.9% -4.7%
T11(14)-D20 R ave 21.7% -7.5%
17-16(8)-D20 165..501p ave 17.8% -11.0%
T11-D20 R beg
72 T16-D12 (c)32..220p (c)32..130p (c) R exp 26.1% !
T12-D18 R pro 25.9% -1.0%
T20-D6 R pro 25.0% -2.7%
16-16-D20 32..165p ave 22.7% -4.8%
20-20-D16 262..501p 131..501p exp 22.1% -5.4%
D20-D16 221..270p pro 22.6% -5.5%
16-16(8)-D20 166..501p beg 18.6% -11.0%
71 T13-D16 (c)32..310p Always 2..501p R exp 26.0% !
T11(14)-D19 (c) ave 25.1% -1.8%
T17-D10 R pro 25.2% -2.7%
70 T14-D14 (c) (c)32..58p exp 27.1% !
T14(11)-D14 49..160p (c)32..40p exp 26.8% -0.2%
T10-D20 32..40p R exp 26.9% -0.3%
T18-D8 33..501p R pro 25.5% -2.6%
14(11)-16-D20 161..200p 33..165p ave 23.4% -4.7%
T20-D5 R exp 23.7% -6.2%
18-20-D16 199..501p exp 22.3% -6.7%
14(11)-16(8)-D20 166..501p beg 20.2% -9.5%
20-B50 R ave
69 T11(14)-D18 (c)32..180p (c)32..167p ave 27.1% !
T15(10)-D12 33..169p exp 27.0% -0.0%
T11-D18 (c)40..40p 181..290p 168..501p R exp 27.1% -0.1%
T15-D12 32..230p R pro 26.8% -0.8%
T19-D6 R pro 26.1% -2.9%
T13-D15 R exp 24.3% -4.8%
T11-4-D16 291..320p exp 22.6% -6.3%
17-20-D16 228..501p pro
68 T12-D16 Always (c)2..501p 151..501p exp 27.2% !
T12(9)-D16 33..122p 32..150p ave 27.1% -0.0%
T16-D10 R pro 27.0% -0.8%
T20-D4 R pro 27.0% -1.3%
T14-D13 (c) ave 26.4% -1.8%
T18-D7 R pro
D18-D16 R pro
67 T9-D20 (c)32..60p (c)32..161p 32..80p exp 28.1% !
T19(7)-D5 (c) ave 26.7% -2.8%
T17-D8 61..340p R pro 25.5% -4.2%
19-16(8)-D16 162..501p exp 25.0% -4.2%
11-16-D20 81..165p ave 23.5% -6.4%
11-16(8)-D20 166..501p beg 19.4% -12.4%
66 T14-D12 32..241p 32..174p (c) R exp 27.9%
T10-D18 (c) (c) 32..60p R ave 28.1% -0.1%
T10(6)-D18 61..100p ave 27.4% -1.1%
T18-D6 R pro 27.1% -2.8%
T16-D9 R exp 26.2% -3.1%
T12-D15 R exp 25.3% -4.6%
B50-D8 242..501p R exp 25.0% -5.7%
14-20-D16 175..501p exp 23.5% -6.0%
D17-D16 R pro
D15-D18 R pro
10-16(8)-D20 166..501p beg
10-16-D20 101..165p ave
65 B50(25)-D20 (c)32..160p (c)32..80p 32..40p R exp 30.8% !
T11-D16 159..310p 81..501p 33..501p R ave 28.1% -2.8%
T19(7)-D4 (c) ave 28.4% -3.5%
T19-D4 R ave 28.6% -3.5%
T15(10)-D10 R ave 27.0% -4.9%
T15-D10 R exp 27.0% -5.2%
64 T16(8)-D8 (c) (c)32..185p (c)32..64p exp 30.3% !
T16-D8 2..501p R pro 30.1% -0.4%
16-8(16)-D20 61..70p ave 26.3% -5.5%
16-16(8)-D16 186..501p exp 26.1% -5.6%
16(8)-8(16)-D20 71..501p beg 25.7% -6.3%
T14-D11 R exp 26.1% -6.7%
D16-D16 R ave
63 T13-D12 (c)32..357p 32..192p 2..501p R exp 27.8%
T17-D6 (c) R pro 28.5% -0.6%
B50(25)-D19 R beg 28.1% -1.3%
T11-D15 R exp 26.1% -3.1%
T19(7)-D3 (c) ave 27.0% -3.7%
15-16(8)-D16 193..320p exp 25.0% -3.8%
13-B50 R beg
62 T14-D10 (c) (c)32..76p R exp 29.4%
T10-D16 2..501p 75..226p R pro 28.2% -1.2%
T10(6)-D16 227..241p exp 27.4% -2.4%
T16(8)-D7 (c) ave 28.1% -2.9%
14-8(16)-D20 2..501p ave 26.1% -4.0%
14-16(8)-D16 242..501p exp 26.0% -4.2%
T12-D13 R exp 26.3% -4.7%
D15-D16 R pro
61 B50(25)-D18 (c)32..120p (c)32..100p (c) R exp 32.3% !
T19(7)-D2 32..64p ave 30.4% -2.6%
T7(19)-D20 R beg 29.9% -2.8%
T15-D8 121..392p 99..120p R ave 29.4% -3.2%
T7-D20 R exp 28.9% -3.9%
T11-D14 R exp 28.1% -5.2%
13-8(16)-D20 63..501p ave 25.8% -7.5%
13-16(8)-D16 119..275p exp 25.7% -7.6%
19-10-D16 276..501p exp 24.9% -8.6%
copyright (c) kari kaitanen 1995
As you see some of these results are quite amazing. Please note that these figures are the "mathematical facts" of the sport and there is nothing I can do to change them - even if I wanted to! In most cases you can however quite easily comprehend the computer's way of thinking and find out why the specific path was recommended.
Please send me your opinion of these pages: kari.kaitanen@vtt.fi.
Yours, Kari