
BUCKY BALL
Instructions for Making One Bucky Ball
Prefabricated triangles are constructed from rolled newspapers
and masking tape. Masking tape is also used to attach
the prefab triangle components.
Two shapes of components are needed, where the lengths of the
sides are S for short and L for long where L = 1.1*S to
make the Bucky Ball any size.
Ten triangles of sides L-L-L need to be made for each
half-dome of the Bucky Ball.
Thirty triangles of sides S-S-L need to be made for each
half-dome of the Bucky Ball.
The 1st row of the Bucky Ball half-dome is made using
5 of the L-L-L Triangles and 5 of the S-S-L Triangles.
With the long side down, alternate the two types
of triangles and attach at the vertices to make
a decagon base.
The 2nd row which will help to secure these base triangles
is made using ten of the S-S-L Triangles with points
down, securely attaching sides as short to short and
long to long.
Continue building by attaching short sides to short sides
and long sides to long sides.
The 3rd row is made using ten of the S-S-L Triangles.
The 4th row is made using five of the L-L-L Triangles.
The 5th row is made using ten of the S-S-L Triangles.
When two half-domes are complete, securely attach these
base to base to make one complete Bucky Ball.
















I have planned many projects throughout the year to tie to the current work, to review and enrich some past work, and to apply mathematical problem solving to financial topics, astronomy, geometry, history, ecology, and the very human activity of game theory. This activity combines applied geometry and proportional thinking to construction engineering with the ultimate use of the product in a game: two geodesic domes will be constructed and joined to form one giant Bucky Ball ( the shape called "Buckminster Fullerine") to be rolled into the goal areas of competing teams. The primary activity is suggested in the the PBS Nova "Building Big" Activity challenge:
The class must work two changes to this project.
These are: 1) groups are to come up with plans for constructing
a much larger dome, and 2) then put two of those domes together
to make the Buckminster Fullerine shaped "Bucky Ball" for use
in a game.
Algebra in our textbook is presented as the manipulation
of transformational equations, with practice organized into problematic
types and themes, and a preponderance of application through the use of
the graphing calculator. 8th graders need more hands-on and socially
interactive learning activities. These students need to review and apply
geometric topics using the mathematics they study now or have earlier
developed into order to sharpen their problem solving skills.
The learning goals were adapted from the Florida Sunshine State
Standards to be assessed in the 8th grade by the Florida Comprehensive
Assessment Test. There are no less than ten benchmark standards for
middle school (M) and high school (H) addressed by this activity:
to use estimation strategies to predict and check
reasonableness of results (M,H);
to solve realworld and mathematical problems
involving estimates (M,H);
to use concrete/graphic models to derive formulas -
perimeter, circumference, ... (M,H);
to use direct and indirect measures to compare metric
or customary units (M);
to understand how the change of a figure in radius,
etc., effects perimeter, etc. (M,H);
to construct, interpret, and use scale drawings to
solve real world problems (M,H);
to solve problems involving units of measure and
convert to ... metric or customary (M);
to understand basic properties of geometric shapes
in two and three dimension (M,H);
to understand geometric concepts ... similarity (M,H);
to represent/apply geometric properties to solve
realworld/mathematical problems (M,H).
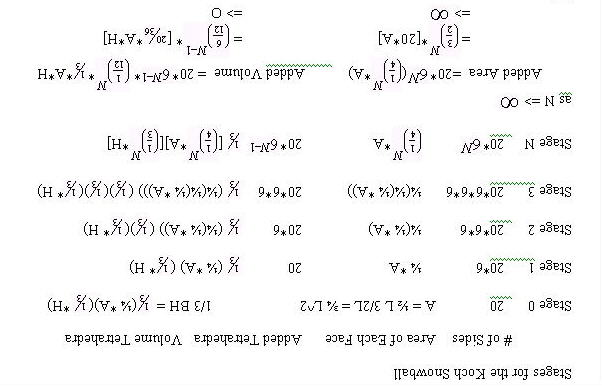
Stages for the Koch Snowflake # of Sides Length of Side Perimeter Area Added Stage 0 3 L 3*L A=(½)(B=L/3)[(H= L)] Stage 1 3*4 L/3 3*4*(L/3) 3*(½)(L/3)[ L] Stage 2 3*4*4 L/(3*3) 3* *(L/ ) 3*(½)(L/ )[ L] Stage 3 3*4*4*4 L/(3*3*3) 3* *(L/ ) 3*(½)(L/ )[ L] Stage N 3* L/ 3* *(L/ ) 3*(½)(L/ )[ L] as N => Added Perimeter = 3* *(L/ ) Added Area = 3*(½)(L/ ) L] = 3* L = [ ] => => O Stages for the Koch Snowball # of Sides Area of Each Face Added Tetrahedra Volume Tetrahedra Stage 0 20 A = ½ L 3/2L = ¾ L^2 1/3 BH = (¼ *A)( *H) Stage 1 20*6 ¼ *A 20 (¼ *A) ( * H) Stage 2 20*6*6 ¼(¼ *A) 20*6 (¼(¼ *A)) ( )( * H) Stage 3 20*6*6*6 ¼(¼(¼ *A)) 20*6*6 (¼(¼(¼ *A))) ( )( )( * H) Stage N 20* *A 20* [ *A][ *H] as N => Added Area = 20* ( *A) Added Volume = 20* * * *A*H = *[20*A] = * [ *A*H] => => O

And one last twist to ponder ... infinite volume within finite time ... infinitely large or infinitismally small black holes ... with or without the fine-structure constraint ???
for the 8th grade Algebra class to remember,
and for everyone else to try for themselves!
