Introductory Astronomy: Study Guide
for Michael A. Seeds, "Foundations of Astronomy" Fourth Edition (1997)
Copyright © 1996 Heather L. Preston and Derek L. Buzasi |
Study Guide for Seeds text, Chapters 1 - 4
You can check out some sample questions on these topics. Use them as flash cards, or tests of your reading comprehension. The multiple choice questions on the exam are mostly variants of these questions (but with a lot of plausible-sounding distractors -- read carefully!)

Chapter 1 -- Scale of the Cosmos, Powers of Ten
This is a good overview chapter, and from it you should take away some sense of the
scales of the Universe. In particular, it would be a good idea to know, or at least
have a sense of, the following scale lengths:
| Earth's diameter | 104 km |
| Sun's diameter | 106 km | |
| Earth's orbit | 108 km | This is one Astronomical Unit (1 A.U.=1.5x108 km) |
| Pluto's orbit | 1010 km | |
| Nearest Stars | 1013 km | One light-year is 9x1012 km. Nearest star = 4.2 ly |
| Open Cluster Diameter | 1015 km | |
| Distance to Galactic Center | 1017 km | |
| Nearest Galaxies | 1019 km | |
| Clusters of Galaxies | 1021 km | |
| Size of Universe | 1023 km | |
Chapter 2: Constellations, the Celestial Sphere, Magnitude Scale
There are 88 official constellations, and about half of these are traditional constellations
such as Pisces and Orion. The newer constellations often have fainter stars, or are
located in the Southern hemisphere. They also tend to have more "modern" names such as Telescopium
, the Telescope.
The brighter stars also have traditional names, such as Sirius
, "The Scorched One" in Arabic, and Aldebaran, "The Follower of the Pleiades." While
beautiful and historic, the traditional names are not very useful in that they tell
us little or nothing about either the location or the brightness of the star. Modern
astronomers therefore use a more rational labelling system, in which Sirius is 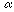 Canis Majoris, "a " meaning that it is the brightest
(next would be b) star in the constellation Canis Major. You should know the first few letters in
the Greek alphabet:
Canis Majoris, "a " meaning that it is the brightest
(next would be b) star in the constellation Canis Major. You should know the first few letters in
the Greek alphabet: 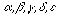 .
.
Magnitude scale
: The Greek astronomer Hipparcos
(or Hipparchus), who lived around 160 - 127 BC, developed a method for classifying
the apparent brightness of stars. He divided all the stars visible to the naked eye
into six classes, which he called magnitudes. The brightest stars were 1st magnitude,
the next brightest 2nd, and so on. The faintest
naked-eye were 6th magnitude.
An important concept to keep in mind when considering the magnitude scale
is that it is logarithmic
rather than linear. This means that a first magnitude star is not 50% brighter than
a second magnitude star, or even twice as bright: it is about 2.5 times as bright.
When modern astronomers adopted the magnitude scale, they modernized it in two ways:
1) A difference of 1 magnitude corresponds to a difference in brightness of 2.512
. This odd number was chosen so that a difference of 5 magnitudes
corresponds to a difference in brightness of 100 times
.
2) The scale was extended to very bright stars (e.g. Sirius, m = -1.4
) and very faint stars (visible in telescopes but not to the naked eye)
Mathematically, the magnitude scale
can be described as follows:
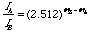 where IA is the intensity (apparent brightness at Earth) of star A and IB of star B. This can also be written as:
where IA is the intensity (apparent brightness at Earth) of star A and IB of star B. This can also be written as:  Note the change in position of the various I's and m's!
Note the change in position of the various I's and m's!
Celestial Sphere
: Ancient (and some not-so-ancient) astronomers believed that the earth was surrounded
by a crystalline sphere, and the stars were attached to this sphere. The sphere rotated
once a day (this kind of motion is called diurnal
), carrying the stars around the earth. We know that this model for the universe is
not actually true, but since it appears to be true, it is still a useful model for
setting up a reference system for finding objects, which we call the celestial sphere
.
From a fixed point on Earth's surface, the sky appears to rotate around an axis that
goes through the north and south celestial poles,
which are located above the earth's north and south poles. The celestial equator
is the projection into the sky of the earth's equator.
The location of the celestial poles and equator in the sky depends on the observer's
latitude.
For an observer at the north pole of the earth, the north celestial pole is always
directly overhead, and the celestial equator lies on the horizon.
As the observer moves southward on the earth's surface, the apparent elevation of
the north celestial pole drops, and that of the equator rises. (See the figure on
page 25 of the text.) For any latitude (except 0 ), some stars will never rise and
set, but will always remain above the horizon: the constellations containing these stars are
called circumpolar
.
Observers in the Northern hemisphere of the earth happen to have a star, Polaris,
located quite close to the north celestial pole. Polaris is
thus known as the North Star,
and its altitude above the horizon is equal to the observer's latitude.
Astronomical Angles:
Angles are measured in degrees, minutes (of arc), and seconds (of arc). There are
360 degrees in a circle, 60 minutes in a degree, and 60 seconds in a minute. Don't
confuse minutes of arc with minutes of time!
Precession:
Hipparcos (the magnitude guy) also compared his observations with those made by a
more ancient culture, the Babylonians, and established that the earth's axis doesn't
point in a constant direction but slowly
circles with time. This phenomenon is similar to what you would observe with a spinning
gyroscope: the axis wobbles in a conical motion, known as precession
. Because the earth is not a perfect sphere but bulges out at the equator, the Moon's
gravity tends to make the earth wobble just like a gyroscope. Since the earth is
very massive, the period for it to complete one wobble is very long: about 26,000 years
. This means that, several thousand years ago, the earth's axis did not point at Polaris,
and no star marked the north celestial pole.
The "Motion" of the Sun:
The Sun has two main apparent motions in the sky. The first is its diurnal rising
and setting which are, as we know, simply reflections of the rotation of the earth.
The second motion is a bit more subtle...
As the earth revolves around the Sun, a process which takes one year
, we see the Sun against different background stars and constellations (or we would,
if the Sun were not so bright!). For example, in January the Sun lies in the same
direction as the constellation Sagittarius (shorthand: the Sun is in the constellation
Sagittarius), while by February the motion of the earth around the Sun has changed things
enough so that the Sun is in the constellation Capricornus.
This apparent path that the Sun sweeps out in the sky over the course of a year is
called the ecliptic.
A band 9 on either side of the ecliptic is called the zodiac,
and the constellations that the Sun passes through during the year are called the
zodiacal constellations.
These are the ones that astrologers use to cast horoscopes.
Another way of thinking of the ecliptic is that it is the plane of the earth's orbit around the Sun
. Most of the other planets (except Pluto) lie in nearly the same plane, and so they
are always found close to the ecliptic, and within the zodiac.
The Seasons
: The earth's axis is not perpendicular to the ecliptic, but is tilted at an angle
of about 23.5 degrees
. This tilt is responsible for our seasons. Look at Figure 2-14 on page 31 in the
text and consider an observer in the Northern hemisphere. In the summer, from his
point of view, the Sun rises early, reaches a point very high in the sky, and sets
late, while in the winter the Sun rises late (or not at all), doesn't get very far over the
horizon, and sets early. The amount of heat delivered to the northern hemisphere
is thus much less in the winter than in the summer for two reasons:
1) The sun spends much less time above the horizon in the winter than in the summer.
2) The angle with which the Sun's rays hit the surface is much less steep in the winter
than in the summer, so the incoming energy is spread out over a much larger area.
See Figure 2-15 on page 32.
There are four locations along the ecliptic that are associated with the seasons.
The vernal equinox
is where the Sun crosses the celestial equator heading north, and is thus the first
day of spring, while the autumnal equinox
is where the Sun crosses the celestial equator heading south, and is thus the first
day of fall. Note that both equinoxes occur where the celestial equator crosses the
ecliptic. Midway between the equinoxes are the solstices
(summer and winter), which define the beginning of summer and winter. Note that all
of this is from the point of view of someone in the northern hemisphere: a person
living in Australia, for example, has all of his seasons reversed with respect to
ours.
Remember that seasons are NOT
due to variations in the earth-Sun distance! (If they were, how would you explain
the reversal of seasons in the southern hemisphere??) In fact, the Sun is at perihelion
(closest approach to the Sun) on about January 2, and at aphelion
(farthest distance from the Sun) in the first week of July. Since the earth's orbit
is nearly circular, these distances only vary by about 2% anyway.
Motion of the Planets:
Planet comes from the Greek work meaningwanderer
. As noted above, all of the planets but Pluto can always be found near the ecliptic.
Remember, "Mother Very Easily Made Jam Sandwiches, Using No Peanutbutter"
for the order of the planets, outward from the Sun: Mercury, Venus, Earth, Mars, Jupiter
Saturn, Uranus, Neptune, Pluto.
Just be sure you remember that Mercury is hot
-- close to the Sun. Also, at the moment, Pluto is closer to the Sun than Neptune,
but things will go back to normal near the end of this century.
As seen from Earth, Mercury and Venus can never move far away from the Sun.This is
because their orbits are smaller than the Earth's. Draw it and see! These two planets,
known as inferior
planets because their orbits lie inside the earth's orbit, can therefore be seen
only around sunrise and sunset, when they are traditionally known as morning stars
or evening stars.
They don't both have to be "morning stars" at the same time, though, since their orbits
have different periods.
F.Y.I. Only (not on test):
Climate and Ice Ages: In the past, the earth has experienced many periods of glaciation,
when the average temperature drops and sheets of ice engulf much of both hemispheres.
The process seems to be periodic, in the following way:
1) There are Ice Age
periods occurring about every 250 million years. The latest started only about 3-5
million years ago. However, just because we are in an Ice Age does not mean that
there are always sheets of ice covering the earth. Within an Ice Age, there are
2) periods of glaciation
, occurring about every 40,000 years and lasting about 20,000 years. Between these
periods, there is an interglacial period
, when the ice sheets melt back. We are living in such a period.
There are many possible explanations for the Ice Age/glaciation phenomenon. The most
plausible are:
1) The changing shape of the earth's orbit. The ellipticity of the earth's orbit varies
with a period of about 93,000 years.
2) Precession of the earth's rotational axis, with a period of about 26,000 years
3) The changing inclination of the earth's axis. The inclination (now 23.5 degrees)
varies from 22 to 28 degrees with a period of about 41,000 years.
4) Passage of the earth and Sun through giant molecular clouds located around our
Galaxy, causing apparent dimming of the Sun. It is an interesting conincidence that
the period of our Sun's revolution around the galactic center is about 250 million
years.
Probably the true cause is a combination of all of the above.=====end of FYI section
=====
Chapter 3: Lunar Phases, Tides, and Eclipses
The phases of the Moon:
The moon orbits the earth at an average distance of about 384,000 km, and its orbit
is elliptical, so that its distance can vary by about 6%. Seen from above the earth's
North pole, the moon orbits counterclockwise (eastward) with a period of about 27.32
days. This is called the sidereal period,
meaning that it is referred to the moon's position with respect to the stars. The
moon takes 29.53 days to circle the sky once and return to the same phase -- 29.53 days is its
synodic period.
The moon's orbit is inclined about 5 degrees with respect to the ecliptic. We say
28 days as shorthand: the phase-based period is 29.5
Study Figure 3-2, which summarizes the lunar phase cycle. Remember that the phase
cycle takes longer than the sidereal period, since the phases are defined with respect
to the Sun. When the moon completes one sidereal period, it is NOT in the same position again with respect to the Sun, since the Earth has moved in its orbit. In fact, the
moon takes a bit more than 2 more days to "catch up" to the Sun, which is why lunar
phases repeat every 29.53 days.
The tides
: Tides are caused by a difference between the gravitational forces acting on different
parts of an object. Consider the earth and the Moon. The side of the earth facing
the moon is pulled on by the moon more than the center of the earth. It therefore
experiences a net force toward the moon (relative to the center of the earth) and will
bulge out in that direction. The far side of the earth is pulled on by the moon less
than the center of the earth. It therefore experiences a net force away from the
moon (relative to the center of the earth) and will bulge out away from the moon. These bulges
are the tides and, as the earth rotates, the bulges occur at different points on
the terrestrial surface. If the Earth's rotation weren't dragging them "forward" (counterclockwise, looking
down from above the N pole), the two bulges would point directly at and away from
the moon.
The tides are most noticeable in the oceans, since the water is free to move, but
the land shows tides as well. Land tides are small, only a few centimeters. If the
earth were a perfect sphere evenly covered by water, tides would occur twice a day
and be the same size everywhere. Since neither of these assumptions is true, tidal ranges
(and even timing) vary from place to place.
The Sun causes tides on the earth as well
. Since the Sun is much more massive than the Moon, its tides would be much larger
if it were close. Fortunately for us(!), it is far away, and so its tides are only
about half the size of lunar tides
.
Solar and lunar tides can act to reinforce one another (when the moon, the Sun, and the earth lie along one line)
, and in this case we get exceptionally large tides, known as spring tides.
On the other hand, the moon and Sun can pull at right angles to one another, and
partly cancel each other. In this case, we get small tides, known as neap tides.
As you can surmise, spring tides don't only occur in the Spring, they occur at full
moon and at new moon (alignment!).
Liewise, neap tides occur at first and third quarters, when the pull is at right angles.
FYI: All that water sloshing back and forth in the oceans creates friction where it
rubs against the ocean floor and the seashores. That friction creates heat, and the
energy to make that heat comes out of the earth's rotation. The earth is therefore
slowing down, and the day is getting longer. This is a slow process (the length of the
day increases by abour 0.001 seconds per century), but the dinosaurs had significantly
shorter days than we do! (end FYI)
Lunar eclipses
: Everything that doesn't glow casts a shadow in space, pointing away from the nearest
glowing body. In the case of the Earth and the Moon, our shadows point away from
the Sun. Such a shadow has two parts:
1) The umbra.
This is the region of total shadow. If you were floating in your spacesuit in the
earth's umbra, you would not be able to see the Sun at all. The umbra of the earth
is about 1.4 million km long and cone-shaped. At the distance of the moon's orbit,
the earth's umbra is about 16000 kilometers in diameter, much larger than the diameter of
the moon.
2) The penumbra.
This is the region of partial shadow. If you were floating in the penumbra, you would
be able to see part of the Sun's surface, a crescent around the edge of the moon.
If the orbit of the moon carries it entirely into the umbra, the moon essentially
disappears (since it shines only by reflected light) and we call the resulting eclipse
total.
The moon takes about an hour from its first contact with the earth's shadow to go
into total eclipse.
Since the earth's shadow points directly away from the sun, the phase of the moon must be full for a lunar eclipse
to happen. Since the moon's orbit is inclined relative to the ecliptic, only some
full moons result in total eclipses, but when they do, the resulting eclipse can
be seen from anywhere on the dark side of the earth.
Normally the moon does not become entirely dark, but glows a deep, dull red, since
the earth's atmosphere bends (refracts
) some sunlight around the edges to light up the moon. If there is a lot of dust in
the atmosphere, this effect is enhanced. The moon is glowing faintly in the light
of a million sunsets.
If the moon only partially enters the umbra, the resulting eclipse is partial.
If it only skims through the penumbra, the eclipse is "penumbral" (which is not very
exciting!).
Solar eclipses
: A solar eclipse occurs when the moon crosses between the earth and the Sun, and
the moon's umbral shadow reaches the earth's surface. The moon's umbral shadow is quite small at the earth's surface, only about 270 km in diameter
. In addition, because of the moon's orbital velocity, the shadow sweeps across the
earth's surface (the path of totality
) at about 1700 km/hr. These two effects combine to make solar eclipses rapid events
(at most 7 minutes) and visible from only a small part of the earth's surface. Of
course, since the moon must pass between the earth and Sun, solar eclipses occur only at new moon
.
If the moon is near apogee
(its farthest distance from the earth), it will not appear large enough in the sky
to cover the Sun, and the eclipse will be annular,
(annulus
means "ring" in Latin) with a thin ring of bright Sun peeking out all the way around
the moon. Only if the moon is near perigee
(closest approach to the earth) can it cover the Sun entirely. If only part of the
Sun is eclipsed, the result is a partial eclipse.
Conditions for an Eclipse:
Nodes:
The moon's orbit is inclined about 5 degrees to the earth's orbit around the Sun
(the ecliptic), and the axis of the moon's orbit precesses. The two points where
the moon's orbit crosses the ecliptic are called nodes,
and since the moon's orbit is precessing, they move with time. To have a true solar
eclipse, we must satisfy the following conditions:
1) The moon must be new, so that it can fall between the earth and the Sun
2) The new moon must be crossing (or very near) a node, so that the Sun is actually
lined up with the Moon. Another way of thinking of this is that the line between
the two nodes (the line of nodes
) must point at the Sun.
3) For a total eclipse, the moon must be near perigee.
An eclipse season
is the period during which everything is sufficiently lined up for an eclipse to
happen. For a solar eclipse, an eclipse season lasts about 32 days
, and any new moon in this period will give rise to a solar eclipse.
The precession of the moon's orbit (unlike Earth's polar precession!) is fast -- it
takes only about 18.6 years to complete a cycle, so people on the earth see the nodes
slipping westward along the ecliptic about 19.4 degrees per year. This means that
eclipse season starts about 19 days earlier every year, and an eclipse year
is only 346.62 days long.
Ancient astronomers predicted eclipses using the Saros cycle.
After one saros cycle of 19 years 11 1/3 days a pattern of eclipses repeats. This
happens because one saros cycle contains 223 lunar months exactly, so after one saros
cycle the moon is back to the same phase it had when the cycle began. But one saros
cycle is also (almost) exactly 19 eclipse years, so the sun will also be back in the
same place in the sky. So if you know when one eclipse happened, you can predict
that another will happen one saros cycle later. Thales
of Miletus was famous for his accurate prediction of eclipses.
Of course, the eclipse that you predict won't be visible from the same part of the
earth, because of that tricky 1/3 day, which means that the earth will have rotated
one-third of the way around from where it was at the start of the cycle. To have
an eclipse recurring at the same part of the earth, you need to wait 3 saros cycles, of 54
years 1 month. This explains why, in any one spot on Earth, it's very rare to see
a solar eclipse!
Chapter 4: History of Astronomy: Greeks and Others
Archaeoastronomy is the study of astronomy as practiced by ancient peoples for whom
there is no surviving written record of observations. The Caracol at Chichen Itza,
Stonehenge, the Bighorn Medicine Wheel, the Moose Mountain Wheel, and the Sun Dagger
in Chaco Canyon are all good examples of archaeoastronomy sites. The stone-age sites
in the New World are not older
than written records of observations in the Old World (including China), so the term
really refers to astronomy carried out at a primitive level of technological advancement
in the local society, as opposed to a specific number of years ago. Thales of Miletus was making observations and predictions centuries before the Moose Mountain Wheel
was built.
In the old world, Thales was followed by several other mathematicians and philosophers.Aristotle
was noteworthy among these for his model of the Universe (actually, the solar system,
although they didn't know the difference at the time) -- with Earth at the center -- called the geocentric
model (wrong) and his observation that the curved shadow in a lunar eclipse meant
the Earth had to be round (right). A very clever fellow named Eratosthenes used a stick and the well
at Syene to determine the Earth's circumference
, about 100 years after Aristotle's death.
The geocentric model failed to explain many subsequent observations of planetary motions
and had to be more and more complicated to get the theoretical positions of planets
at any given time to agree with the observations. The kludge-meister of the geocentric model was Ptolemy
(a.k.a. Ptolemaeus), who had a fabulously complex geocentric model with epicycles, deferents and equants
. The Ptolemaic system ruled astronomy for more than 1300 years! Copernicus
finally came out with his revolutionary
book (de Revolutionibus Orbium Coelestium
) placing the Sun at the center. This heliocentric
view, because the fundamental assumption was correct, finally overthrew the geocentric
system. But not without a fight!
Galileo
took up the banner where dead Copernicus had dropped it, and used a new Dutch invention
-- the telescope
-- to look at heavenly objects. He discovered the four large inner moons of Jupiter (called the Galilean moons in his honor), and observed
the phases of Venus.
This observation really got him into hot water with the Holy Roman Empire, because
they were sticking with the geocentric theory, and you can't have phases such as
a gibbous Venus and
a crescent Venus unless Venus goes around
the Sun (work it out with a ball and a light source). He also had the cheek to observe
sunspots on the Sun and craters on the Moon, evidences of imperfection from the point
of view of that time (when many many people had terrible skin due to smallpox; they had a real smooth-skin fixation and considered smoothness evidence of perfection.
Heavenly bodies were supposed to be perfect).
Tycho Brahe
, in Denmark, had been naked-eye observing for many years before Galileo was born,
and his work (moved to Prague by a change in patrons) was turned over to his assistant,
Johannes Kepler
, on his death. Shortly after that, the telescope was invented, and so Kepler really
went into high gear. He observed the motions of the planets even more accurately
than Tycho had been able to, but he had all of Tycho's data giving him a long timeline
to work with. He came up with Kepler's three laws of planetary motion
:
- Orbits are ellipses
, with the central body at one focus of the ellipse.
- When the orbiting body is closer to the central body it moves faster. At the far
end of its orbit it moves slower. A line from the central body to the orbiting body
would sweep out equal pie-slice-shaped areas in equal times.
- P2=a3
-- He saved the best for last. P is the period of the planet's orbit around the Sun
(in years), and a is the semi-major axis of the ellipse (the average distance from
the Sun), in A.U. Newton later generalized this equation so as to apply it to any
system, not just the Solar system.
Office: 3019 Nevins Hall
Phone: (912) 333-5752
You can reach me by e-mail at: hpreston@valdosta.peachnet.edu
Go to Heather's Home Page

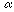 Canis Majoris, "a " meaning that it is the brightest
(next would be b) star in the constellation Canis Major. You should know the first few letters in
the Greek alphabet:
Canis Majoris, "a " meaning that it is the brightest
(next would be b) star in the constellation Canis Major. You should know the first few letters in
the Greek alphabet: 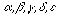 .
.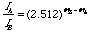 where IA is the intensity (apparent brightness at Earth) of star A and IB of star B. This can also be written as:
where IA is the intensity (apparent brightness at Earth) of star A and IB of star B. This can also be written as:  Note the change in position of the various I's and m's!
Note the change in position of the various I's and m's!