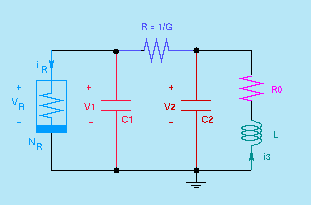

Chua's circuit consists of two linear capacitors, two linear resistors, one linear inductor and a nonlinear resistor. By varying the various circuit parameters, we obtain complicated nonlinear and chaotic phenomena. Let us consider the case where we vary the conductance G of the resistor R and keep the other components fixed. In particular, we choose L = 18mH, R0=12.5 Ohms, C2 = 100nF, C1 = 10nF. The nonlinear resistor (Chua's diode) is chosen to have a piecewiselinear V-I characteristic of the form:Fig. 1
_ | Gb*v + Ga-Gb if v > 1 i = -| Ga*v if |v| < 1 | Gb*v + Gb-Ga if v < -1 -with Ga = -0.75757mS, and Gb = -0.40909mS.
Starting from G = 500e-6, the circuit is stable, and all trajectories converge towards one of the two stable equilibrium points. As G is increased, a limit cycle appears due to a Hopf-like bifurcation. In order to observe the period-doubling route to chaos, move the slider below to change G.
At the end of the period-doubling bifurcations, we observe a chaotic attractor. Because of symmetry, there exists a twin attractor lying in symmetrical position with respect the the origin. As G is further increased, these two chaotic attractors collide and form a "double scroll" chaotic attractor.
After normalization, the state equations can be given by:
.
x = a*(y-x-f(x))
.
y = x-y+z
.
z = -b*y - c*z
Back to introduction.