Then, the sequence families for binding of different transcription factors can be very similar or overlap each other. In other words, the same target sequence can be recognized by two factors, especially if they have antagonistic action on the same targeting gene. These two characteristics are necessary and sufficient to ensure the recruiting phenomenon.
Consider the following oversimplified example. Let us have an exponentially decaying gradient of morphogen M along the longitudinal axis of a rudiment. (We consider the one-dimensional case, for simplicity.) Assume that M is a site-specific transcription factor and its target is gene X. M has affinity to the CATAAT-like family of sequences and the regulatory region of the X has two or three such sites. Assume that M acts on activity of the X in a concentration-dependent manner: low concentrations of M activate X, while high concentrations inhibit X. As a result, we obtain a bell-shaped concentration profile of the X product along the exponentially decaying gradient of M.
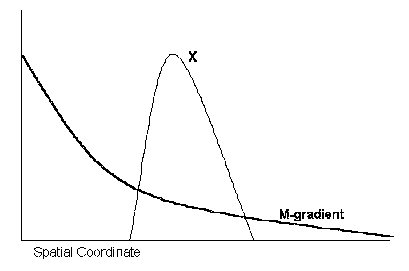
Figure 2 Spike-like concentration profile of the X product along exponential decaying gradient of M.
For example, the Kruppel gene is believed to be activated by HB proteins (See previous section). A rate law comprising this could be:
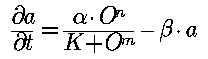
where a is the Kr product concentration and O is the HB protein concentration. This is a generalized Hill-type rate law that would yield concentration-dependent regulation (if m>n): cooperative activation for small values of O and inhibition for large values of O.
Then assume that two new genes, A and R, appear in the genotype, as a result of duplications. For simplicity assume that both new genes also are activated by M, but through pure activation. This yields S-shaped (not bell-shaped) profiles of the A and R products along the M-gradient. The R-gradient is shifted, respective to A-gradient, to higher edge of the M gradient. And last, assume that the A-product is a pure activator for target genes, while the R-product is a repressor. Targeting sequences for the A product include AATAAT, while the target sequence for R includes AATAA. Then, single point mutation in one of the M-binding sites of the X-gene regulatory region, such as CATAAT => AATAAT, turns the X gene into a target for the A- and R-products (apart from M). (We are omitting details of coactivation by different activators that will be considered in the next section.)
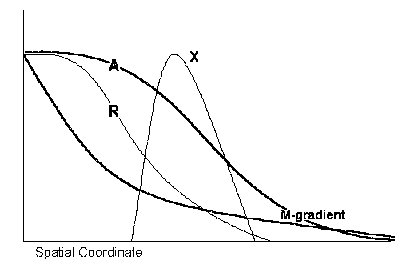
Figure 3. S-shaped gradients of Activator and Repressor, (along the axis and the M-gradient). Action of A-product and R-product on the X mimics action of the M.
Now the main idea became clear. Action of the activator A-product and the repressor R-product mimics action of the M. Namely, A-product gives an S-shaped gradient of the X, (along the axis and the M-gradient) similar to its concentration profile, while the R-product represses X-production at the higher edge of the M gradient. Finally we obtain the same bell-shaped concentration profile, as in the case of the M-gradient action alone.
Hence we achieve the recruitment of two new members into the simple cascade of X-gene activation. Finally the M activates A- and R-genes and coaction of A- and R-products (plus M action) yields a pattern of X activation similar to the initial type of the X pattern. As we can see, the same result is achieved by action of a more complicated gene network (without an apparent selective advantage of the new network).
Then, it is obvious that appropriate mutation in one of M-binding sites of X-gene occurs with very low probability. But if we consider such large populations, as found for many insects, and large amounts of evolutionary time, then the number of mutants with enlarged networks will slowly rise from generation to generation.