

|
Operating Instructions for Models K and L. |
The following pages contain
examples of various calcula-
tions showing the best method
of dealing with them on the
Otis King's Patent Calculator
| CARBIC LIMITED, 51, Holborn Viaduct, LONDON, E.C. 1. |
OTIS KING'S PATENT CALCULATOR
The Calculator consists of two metal tubes, the smaller (cylinder) being free to rotate and slide within the larger (holder). Spiral logarithmic scales are mounted on each of these tubes, while a third tube mounted on the holder forms a tubular cursor carrying at each end an arrow, which can be set to any mark, or to which any mark can be set.
The three parts of the instrument are inseparable, and being made of brass throughout there is no possibility of their warping or being affected in any other way by climatic conditions. At the top of the holder there are three metal sprags, which hold the cursor in position, and at the same time prevent it touching the scales. The bottom end of the cylinder is covered with velvet, which permits it to slide and rotate freely within the holder, but at the same time holds it firmly in position, and thus prevents any chafing between the two tubes. The metal parts are carefully machined, and all exposed parts are heavily nickelled.
The scales are reproduced photographically on a specially prepared material, which has an extremely hard waterproof surface.
In the following pages will be found detailed instructions in the use of these instruments for all kinds of technical and commercial calculations, with details of the scales carried on each instrument. Rules for determining the position of the decimal point will be found on pages 10 and 11.

TO CLEAN SCALES
The scales may be cleaned from time to time by rubbing lightly with a clean piece of cloth dipped in paraffin or petrol. Allow to dry before using again.
The Calculator consists of the following parts :-
| (1) | THE HOLDER | } | on which are mounted spiral logarithmic scales. |
| (2) | THE CYLINDER | ||
| (3) | THE INDICATOR (or cursor) on which two arrows are engraved. | ||
The following sequence of movements should always be adhered to in operating the Instrument :-
| MOVEMENT 1.- | Take the HOLDER in the left hand, and open Instrument to full extent. Move INDICATOR to set bottom arrow. |
| MOVEMENT 2.- | Move CYLINDER so that number on scale is set to top arrow (not the arrow to the number). |
| MOVEMENT 3.- | Move INDICATOR to set either arrow as required - the companion arrow will then point to the answer. (See examples on following pages). |
DETAILS OF SCALES.
Model K.
| By | 001 | from | 1 | to | 1 .1 | By | 005 | from | 2 | to | 4 | ||
| ,, | 002 | ,, | 1 .1 | ,, | 2 | ,, | 01 | ,, | 4 | ,, | 10. |
THE TOP SCALE (ON CYLINDER) consists of two scales in series which are identical in all respects with the above. The point at which these two scales meet half way up the cylinder is denoted by the word "one," and is referred to in the following pages as the "middle unity."
This double scale reduces the number of operations involved in a series of calculations because it ensures that every incoming factor is at a place to which the Indicator can be immediately set.
Model L.
THE BOTTOM SCALE (ON HOLDER) is identical with the Bottom Scale on Model "K" (see page 4).
THE TOP SCALE (CYLINDER). - Instead of the double logarithmic scale as on Model "K," the lower half consists of a scale from 0 to 10, graduated in 2,000 equal divisions of .005 each. This is used in conjunction with the scale on Holder to obtain the logarithm of any required number.
The Upper Half of the Scale is identical with that on the Cylinder on Model "K" from middle unity upwards, and is used in conjunction with Scale on Holder for multiplication, division, &c.
EXAMPLES.
The following instructions apply to both Models K and L :-
Multiplication.
Example :- Multiply
16 .5 by 14.
Set bottom arrow to 16 .5
(multiplicand). Set middle unity to top arrow. Move top arrow to
14 (multiplier) and read answer at bottom arrow :-
16 .5x14=231.
Division.
Example :- Divide 16 .5 by
3 .3.
Set bottom arrow to 16 .5
(dividend). Set 3 .3 (divisor) to
top arrow. Move top arrow to available unity. Read answer at
bottom arrow :-
Fractions into Decimals and vice versa
Example :- What is the decimal equivalent of 7/8 ?
Set bottom arrow to 7 (numerator). Set 8 (denominator) to top
arrow. Move top arrow to available unity. Read answer at
bottom arrow :- 7/8= .8750.
What is the fractional equivalent of
.8750 ?
Set bottom arrow to .8750
(decimal figure). Set middle unity to top arrow. Move top
arrow to any denominator (say 8). Read numerator at bottom
arrow. Thus, .8750=7/8.
Proportions.
Example :- Solve
12 : 7 :: 16 : ?
Set bottom arrow to 12. Set 7 to top arrow. Move bottom
arrow to 16. Read answer at top arrow :-
12 : 7 :: 16 : 9 .333.
To Solve Percentage Problems.
Set bottom arrow to capital amount or quantity. Set middle unity
to top arrow. The Instrument is now set to solve percentage problems
involving % ON, % OFF and % OF.
| Example I. :- What is 5% | (a) | of 162 ? |
| (b) | off 162 ? | |
| (c) | on 162 ? |
Constant Factors.
In cases where one factor is repeated throughout a series of problems,
the instrument may be set to the constant term, so that the answer may be found
by one movement of the indicator only.
Example :- What is 5% on 120, 136, 53, 42,
12 .5,
76 .4 ?
Set bottom arrow to 105 (100+5%). Set middle index to top
arrow. (Note :- The instrument is now set for any
problems involving a calculation of 5% on). Move top arrow to
120. Read answer at bottom arrow, viz., 126. Now move top arrow to
136. Read answer at bottom arrow, viz.,
142 .8, and so on right
through the series.
Where involved expressions occur above or below the line, the Otis King's Calculators offer valuable advantages over the ordinary slide rule, which, even if engraved with log-log scales cannot solve the following, whereas model L will give all powers and roots, fractional or otherwise, of all numbers without limit, and solve any expression, however extended. The following expression is given as an example :-
1. 0083 .1
x
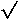 63 636 x 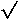 260000 260000 | = .25.. |
| 1. 025
x
3.98
x 4000 6 x 12 .11 x 900 .1 |
To LOGARIZE -
(i.e., find the logarithm representing a number).
Set bottom arrow to bottom unity. Set "0" of lower top scale to top
arrow. Set bottom arrow to number (antilogarithm),
and read mantissa at top arrow.
To DELOGARIZE -
(i.e., to find the number represented by a logarithm).
Set bottom arrow to bottom unity. Set "0" of lower top scale to
top arrow. Set top arrow to mantissa. Read antilogarithm (number)
at bottom arrow. *
To ascertain any Power or Root of any number.
| POWERS. | ROOTS. | |||
| The power sought is the delogarized product of the logarized number multiplied by the figures of the index of the power. | The root sought is the delogarized quotient of the logarized number divided by the figures of the index of the root. | |||
| Example :- What is
4.12.2 Log. of 4.1 = 0.6128. 0.6128x2.2 = 1.348. Antilog. of 1.348 = 22.29. Therefore 4.12.2 = 22.29. |
Example :- What is
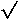 9.1 9.1Log. of 9.1 = 0.9590. 0.9590÷3.1 = 0.3093. Antilog. of 0.3093 = 2.038. Therefore 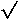 9.1
= 2.038 9.1
= 2.038 |
|||
TO DETERMINE POSITION OF DECIMAL POINT.
| have | 5430000, +7 |
674, +3, |
81.2, +2, |
7 .82, +1, |
0 .45, +0, |
0.0421, -1, |
0.00675; -2 |
places |
DIVISION.
Calculations involving Multiplication and Division.
| RULE III.- | Two methods may be used in working out complex problems involving both multiplication and division. They are :- | |
| (1) | Taking numerator and denominator alternately. | |
| (2) | Taking all the numerators first and then dividing consecutively by the denominators. | |
| Of these two methods, only the latter can be used if the position of the decimal point is required. If the other is used, the decimal point must be found by inspection. | ||
| First multiply consecutively the series of factors in the numerator and then divide consecutively by the factors of the denominator. | ||
| Take the algebraic sum of the places in the factors of the denominator from the algebraic sum of the places in the factors of the numerator, and to this result add the algebraic sum of the results obtained from the application of Rules I. and II. to the several steps of the problem. | ||
| Example: | 432 x 32.4
x 0.0217 x 0.98 0.00000621 x 412000 x 0.175 x 4.71 |
= 141.14... | |||||||
| No. | of | places | in | factors | of | numerator | = 3+2+(-1)+0 | = +4. | |
| ,, | ,, | ,, | ,, | ,, | ,, | denominator | = -5+6+0+1 | = +2.
|
|
| Difference | = +2. | ||||||||
| Results of various steps in calculation | = -1+1+1 | = +1. |
|||||||
| Number of places in answer | = +3. | ||||||||
| Answer = 141.14.... | |||||||||