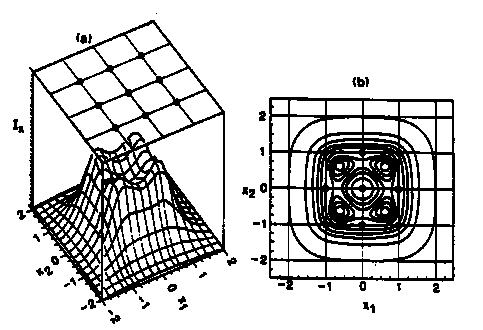
nsider the information function for the

32

factorial design.

Writing

this is

=

nz' (X'X)-1z
? ?
? ? ?

?

-1

=

5

-4.5(x1

2

+x2
2)

+4.5(x1

2

+x2)2
2

-6.75x2
1x2

2

-1

ich is graphed in Fig. a and has its contours plotted in Fig. b.

It will be seen

t the design generates four pockets of high information which seemingly hav

le to do with the needs of an experimenter.

Rather than attempt to generalize

property of orthogonality to seond order, we shall instead generalize the

perty of rotatability.

We shall see that it is possible to choose designs of

cond and higher orders for which the information contours are spherical.

uivalently, these rotatable designs have the property that the varainces and

variances of the effects remain unaffected by rotation.

One such rotatable

sign for two factors, consisting of eight points evenly distributed on a circle wi

r added center points is together with its information function.

Informationsurface for 32factorial usedas a second-orderdesign.

The correspondinginformationcontours.

e figuresshowhowthe variancesof the second-ordertermsand the

rrelationsbetweenthemchange as the 32designis rotatedthrough an angle

(For simplicity,we sets2= 1

here.)Thus, although this 32second-order

sign is orthogonal(in the sensedescribed)in its initial orientation,it losesthis

perty on rotation,unlike the first-orderdesign.In viewof this, it would seem

st sensibleto choose,if possible,a second-orderdesignfor which the

riancesand covariancesof the estimatesstay constantas the designis

ated.